Advertisements
Advertisements
प्रश्न
Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2CD, find the ratio of the areas of triangles AOB and COD.
उत्तर
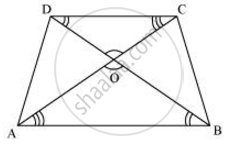
Since AB || CD,
∴ ∠OAB = ∠OCD and ∠OBA = ∠ODC (Alternate interior angles)
In ΔAOB and ΔCOD,
∠AOB = ∠COD (Vertically opposite angles)
∠OAB = ∠OCD (Alternate interior angles)
∠OBA = ∠ODC (Alternate interior angles)
∴ ΔAOB ∼ ΔCOD (By AAA similarity criterion)
`:.(ar(ΔAOB))/(ar(COD)) = ((AB)/(CD))^2`
Since AB = 2 CD
`:. (ar(triangleAOB))/(ar(triangleCOD)) =((2CD)/(CD))^2 =4/1=4:1`
APPEARS IN
संबंधित प्रश्न
In two similar triangles ABC and PQR, if their corresponding altitudes AD and PS are in the ratio 4 : 9, find the ratio of the areas of ∆ABC and ∆PQR
In figure below ΔACB ~ ΔAPQ. If BC = 10 cm, PQ = 5 cm, BA = 6.5 cm and AP = 2.8 cm,
find CA and AQ. Also, find the area (ΔACB): area (ΔAPQ)

In Figure, DE || BC If DE = 4 cm, BC = 6 cm and Area (ΔADE) = 16 cm2, find the area of ΔABC.

ABCD is a trapezium in which AB || CD. The diagonals AC and BD intersect at O. Prove that: (i) ΔAOB and ΔCOD (ii) If OA = 6 cm, OC = 8 cm,
Find:(a) `("area"(triangleAOB))/("area"(triangleCOD))`
(b) `("area"(triangleAOD))/("area"(triangleCOD))`
AD is an altitude of an equilateral triangle ABC. On AD as base, another equilateral triangle ADE is constructed. Prove that Area (ΔADE): Area (ΔABC) = 3: 4
The ratio of corresponding sides of similar triangles is 3 : 5, then find the ratio of their areas.
If ∆XYZ ~ ∆PQR and A(∆XYZ) = 25 cm2, A(∆PQR) = 4 cm2 then XY : PQ = ?
In the given figure DE || AC which of the following is true?
O is a point on side PQ of a APQR such that PO = QO = RO, then ______.
In the given figure, D is the mid-point of BC, then the value of `(coty^circ)/(cotx^circ)` is ______.