Advertisements
Advertisements
प्रश्न
Draw a parallelogram ABCD in which BC = 5 cm, AB = 3 cm and ∠ABC = 60°, divide it into triangles BCD and ABD by the diagonal BD. Construct the triangle BD' C' similar to ∆BDC with scale factor `4/3`. Draw the line segment D'A' parallel to DA where A' lies on extended side BA. Is A'BC'D' a parallelogram?
उत्तर
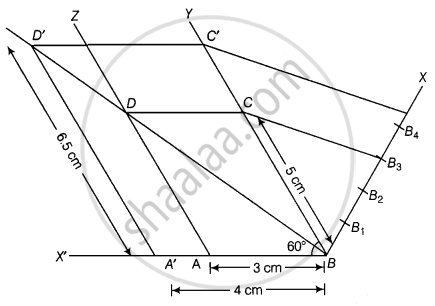
Steps of construction
- Draw a line segment AB = 3 cm.
- Now, draw a ray BY making an acute ∠ABY = 60°.
- With B as centre and radius equal to 5 cm draw an arc cut the point C on BY.
- Again draw a ray AZ making an acute ∠ZAX’ = 60°. ...[∴ BY || AZ, ∴ ∠YBX’ = ZAX’ = 60°]
- With A as centre and radius equal to 5 cm draw an arc cut the point D on AZ.
- Now, join CD and finally make a parallelogram ABCD.
- Join BD, which is a diagonal of parallelogram ABCD.
- From B draw any ray BX downwards making an acute ∠CBX.
- Locate 4 points B1, B2, B3, B4 on BX, such that BB1 = B1B2 = B2B3 = B3B4.
- Join B4C and from B3C draw a line B4C’ || B3C intersecting the extended line segment BC at C’.
- From point C’ draw C’D’ || CD intersecting the extended line segment BD at D’. Then, AD’BC’ is the required triangle whose sides are `4/3` of the corresponding sides of ΔDBC.
- Now draw a line segment D’A’ parallel to DA, where A’ lies on extended side BA i.e., ray BX’.
- Finally, we observe that A’BCD’ is a parallelogram in which A’D’ = 6.5 cm A’B = 4 cm and ∠A’BD’ = 60° divide it into triangles BC'D’ and A’BD’ by the diagonal BD.
APPEARS IN
संबंधित प्रश्न
Divide a line segment of length 14 cm internally in the ratio 2 : 5. Also, justify your construction.
Draw a right triangle ABC in which AC = AB = 4.5 cm and ∠A = 90°. Draw a triangle similar to ΔABC with its sides equal to (5/4)th of the corresponding sides of ΔABC.
Draw a line segment of length 7.6 cm and divide it in the ratio 5:8. Measure the two parts.
Construct a right triangle in which the sides, (other than the hypotenuse) are of length 6 cm and 8 cm. Then construct another triangle, whose sides are `3/5` times the corresponding sides of the given triangle.
Points P and Q trisect the line segment joining the points A(−2, 0) and B(0, 8) such that P is near to A. Find the coordinates of points P and Q.
ΔRHP ~ ΔNED, In ΔNED, NE = 7 cm. ∠D = 30°, ∠N = 20°, `"HP"/"ED" = 4/5`, then construct ΔRHP and ∆NED
If the point P (6, 7) divides the segment joining A(8, 9) and B(1, 2) in some ratio, find that ratio
Solution:
Point P divides segment AB in the ratio m: n.
A(8, 9) = (x1, y1), B(1, 2 ) = (x2, y2) and P(6, 7) = (x, y)
Using Section formula of internal division,
∴ 7 = `("m"(square) - "n"(9))/("m" + "n")`
∴ 7m + 7n = `square` + 9n
∴ 7m – `square` = 9n – `square`
∴ `square` = 2n
∴ `"m"/"n" = square`
If the perpendicular distance between AP is given, which vertices of the similar triangle would you find first?
If you need to construct a triangle with point P as one of its vertices, which is the angle that you need to construct a side of the triangle?

Draw a triangle ABC in which AB = 4 cm, BC = 6 cm and AC = 9 cm. Construct a triangle similar to ∆ABC with scale factor `3/2`. Justify the construction. Are the two triangles congruent? Note that all the three angles and two sides of the two triangles are equal.
