Advertisements
Advertisements
प्रश्न
Evaluate:
`(5 cos^2 60^circ + 4 sec^2 30^circ - tan^2 45^circ)/(sin^2 30^circ + cos^2 30^circ)`
उत्तर
`(5 cos^2 60^circ + 4 sec^2 30^circ - tan^2 45^circ)/(sin^2 30^circ + cos^2 30^circ)`
=`(5(1/2)^2+4(2/sqrt(3))^2-(1)^2)/((1/2)^2+(sqrt(3)/2)^2)`
=`((5/4 + (4xx4)/3-1))/((1/4+3/4)`
=`((5/4+16/3-1))/((4/4))`
=`(((15 + 64 - 12)/12))/((4/4))`
=`((67/12))/((1))`
=`67/12`
APPEARS IN
संबंधित प्रश्न
If ∠A and ∠P are acute angles such that tan A = tan P, then show that ∠A = ∠P.
If θ = 30° verify that `sin 2theta = (2 tan theta)/(1 + tan^2 theta)`
In a ΔABC , ∠B = 90° , AB = 12 cm and BC = 5 cm Find
(i) cos A (ii) cosec A (iii) cos C (iv) cosec C
In triangle ABC, AD is perpendicular to BC. sin B = 0.8, BD = 9 cm and tan C = 1.
Find the length of AB, AD, AC, and DC.
Given q tan A = p, find the value of:
`("p" sin "A" – "q" cos "A")/("p" sin "A" + "q" cos "A")`.
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of `(1)/("sin"^2 x) - (1)/("tan"^2 x)`
In the given figure, ΔABC is right angled at B.AD divides BC in the ratio 1 : 2. Find
(i) `("tan"∠"BAC")/("tan"∠"BAD")` (ii) `("cot"∠"BAC")/("cot"∠"BAD")`
From the given figure, find the values of sec B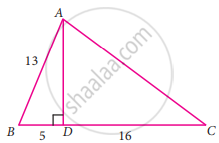
If 2 cos θ = `sqrt(3)`, then find all the trigonometric ratios of angle θ
Assertion (A): For 0 < 0 ≤ 90°, cosec θ – cot θ and cosec θ + cot θ are reciprocal of each other.
Reason (R): cot2 θ – cosec2 θ = 1.
