Advertisements
Advertisements
Question
Evaluate:
`(5 cos^2 60^circ + 4 sec^2 30^circ - tan^2 45^circ)/(sin^2 30^circ + cos^2 30^circ)`
Solution
`(5 cos^2 60^circ + 4 sec^2 30^circ - tan^2 45^circ)/(sin^2 30^circ + cos^2 30^circ)`
=`(5(1/2)^2+4(2/sqrt(3))^2-(1)^2)/((1/2)^2+(sqrt(3)/2)^2)`
=`((5/4 + (4xx4)/3-1))/((1/4+3/4)`
=`((5/4+16/3-1))/((4/4))`
=`(((15 + 64 - 12)/12))/((4/4))`
=`((67/12))/((1))`
=`67/12`
APPEARS IN
RELATED QUESTIONS
Given 15 cot A = 8. Find sin A and sec A.
If x = cosec A +cos A and y = cosec A – cos A then prove that `(2/(x+y))^2 + ((x-y)/2)^2` = 1
Evaluate:
`(sin30°)/(cos 45°)+(cot45°)/(sec60° )- (sin60°)/(tan45°)+(cos30°)/(sin90°)`
`(cos 28°)/(sin 62°)` = ?
In the given figure;
BC = 15 cm and sin B = `(4)/(5)`
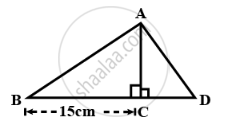
- Calculate the measure of AB and AC.
- Now, if tan ∠ADC = 1; calculate the measures of CD and AD.
Also, show that: tan2B - `1/cos^2 "B" = – 1 .`
If cosB = `(1)/(3)` and ∠C = 90°, find sin A, and B and cot A.
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cos C
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of sin x
If cosec θ = `(29)/(20)`, find the value of: cosec θ - `(1)/("cot" θ)`
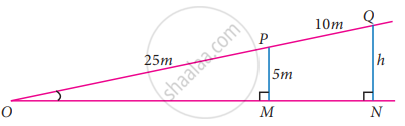
A boy standing at a point O finds his kite flying at a point P with distance OP = 25 m. It is at a height of 5 m from the ground. When the thread is extended by 10 m from P, it reaches a point Q. What will be the height QN of the kite from the ground? (use trigonometric ratios)