English Medium
Academic Year: 2022-2023
Date & Time: 21st March 2023, 10:30 am
Duration: 3h
Advertisements
General Instructions:
- This question paper contains 38 questions.
All questions are compulsory. - This Question Paper is divided into FIVE Sections - Section A, B, C, D, and E.
- In Section - A question number 1 to 18 are Multiple Choice Questions (MCQs) and question number 19 & 20 are Assertion Reason based question of 1 mark each.
- In Section - B questions number 21 to 25 are Very Short-Answer-I (SA-I) type questions of 2 marks each.
- In Section - C questions number 26 to 31 are Short Answer-II (SA-II) type questions carrying 3 marks each.
- In Section - D questions number 32 to 35 are Long Answer (LA) type questions carrying 5 marks each.
- In Section - E questions number 36 to 38 are Case Study/Passage based integrated units and assessment questions carrying 4
marks each. Internal choice is provided in 2 marks question in each case-study. - There is no overall choice. However, an internal choice has been provided in 2 questions in Section - B, 2 questions in Section - C, 2 questions in Section - D, and 3 questions in Section - E.
- Draw neat figures wherever required. Take π = `22/7` wherever required if not stated.
- Use of calculator is NOT allowed.
Which of the following is true for all values of θ (0° ≤ θ ≤ 90°)?
cos2 θ – sin2 θ = 1
cosec2 θ – sec2 θ = 1
sec2 θ – tan2 θ = 1
cot2 θ – tan2 θ = 1
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
If k + 2, 4k – 6 and 3k – 2 are three consecutive terms of an A.P., then the value of k is ______.
3
–3
4
–4
Chapter: [0.024] Arithmetic Progressions
The next term of the A.P. : `sqrt(6), sqrt(24), sqrt(54)` is ______.
`sqrt(60)`
`sqrt(96)`
`sqrt(72)`
`sqrt(216)`
Chapter: [0.024] Arithmetic Progressions
The distance of the point (–1, 7) from x-axis is ______.
–1
7
6
`sqrt(50)`
Chapter: [0.031] Lines (In Two-dimensions) [0.031] Lines (In Two-dimensions)
What is the area of a semi-circle of diameter ‘d’?
`1/16 πd^2`
`1/4 πd^2`
`1/8 πd^2`
`1/2 πd^2`
Chapter: [0.042] Circles
The empirical relation between the mode, median and mean of a distribution is ______.
Mode = 3 Median – 2 Mean
Mode = 3 Mean – 2 Median
Mode = 2 Median – 3 Mean
Mode = 2 Mean – 3 Median
Chapter: [0.071] Statistics
The pair of linear equations 2x = 5y + 6 and 15y = 6x – 18 represents two lines which are ______.
intersecting
parallel
coincident
either intersecting or parallel
Chapter:
If α and β are the zeroes of the polynomial x2 – 1, then the value of (α + β) is ______.
2
1
–1
0
Chapter: [0.021] Polynomials
A pole 6 m high casts a shadow `2sqrt(3)` m long on the ground, then the Sun’s elevation is ______.
60°
45°
30°
90°
Chapter: [0.053] Some Applications of Trigonometry
sec θ when expressed in term of cot θ, is equal to ______.
`(1 + cot^2 θ)/cotθ`
`sqrt(1 + cot^2 θ)`
`sqrt(1 + cot^2 θ)/cotθ`
`sqrt(1 - cot^2 θ)/cotθ`
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
For the following distribution:
| Class | 0 – 5 | 5 – 10 | 10 – 15 | 15 – 20 | 20 – 25 |
| Frequency | 10 | 15 | 12 | 20 | 9 |
The sum of lower limits of the median class and modal class is:
15
25
30
35
Chapter: [0.071] Statistics
The length of tangent drawn to a circle of radius 9 cm from a point 41 cm from the centre is ______.
40 cm
9 cm
41 cm
50 cm
Chapter: [0.042] Circles
In figure, if O is the centre of a circle PQ is a chord and the tangent PR at P makes an angle of 50° with PQ, then ∠POQ is equal to ______.
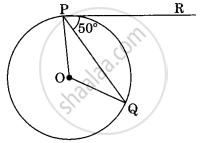
100°
80°
90°
75°
Chapter: [0.042] Circles
A bag contains 5 red balls and n green balls. If the probability of drawing a green ball is three times that of a red ball, then the value of n is ______.
18
15
10
20
Chapter: [0.07200000000000001] Probability
In figure, AT is a tangent to the circle with centre O such that OT = 4 cm and ∠OTA = 30°. Then AT is equal to ______.

4 cm
2 cm
`2sqrt3` cm
`4sqrt3` cm
Chapter: [0.042] Circles
In ΔABC, PQ || BC. If PB = 6 cm, AP = 4 cm, AQ = 8 cm, find the length of AC.

12 cm
20 cm
6 cm
14 cm
Chapter: [0.040999999999999995] Triangles [0.040999999999999995] Triangles [0.040999999999999995] Triangles
If α, β are the zeroes of the polynomial p(x) = 4x2 – 3x – 7, then `(1/α + 1/β)` is equal to ______.
`7/3`
`(-7)/3`
`3/7`
`(-3)/7`
Chapter: [0.021] Polynomials
Advertisements
A card is drawn at random from a well-shuffled pack of 52 cards. The probability that the card drawn is not an ace is ______.
`1/13`
`9/13`
`4/13`
`12/13`
Chapter: [0.07200000000000001] Probability
Assertion (A): The probability that a leap year has 53 Sundays is `2/7`.
Reason (R): The probability that a non-leap year has 53 Sundays is `5/7`.
Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).
Both Assertion (A) and Reason (R) are true and Reason (R) is not the correct explanation of Assertion (A).
Assertion (A) is true but Reason (R) is false.
Assertion (A) is false but Reason (R) is true.
Chapter: [0.07200000000000001] Probability
Assertion (A): a, b, c are in A.P. if and only if 2b = a + c.
Reason (R): The sum of first n odd natural numbers is n2.
Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).
Both Assertion (A) and Reason (R) are true and Reason (R) is not the correct explanation of Assertion (A).
Assertion (A) is true but Reason (R) is false.
Assertion (A) is false but Reason (R) is true.
Chapter: [0.024] Arithmetic Progressions
Evaluate: `5/(cot^2 30^circ) + 1/(sin^2 60^circ) - cot^2 45^circ + 2 sin^2 90^circ`.
Chapter: [0.051] Introduction to Trigonometry
If θ is an acute angle and sin θ = cos θ, find the value of tan2 θ + cot2 θ – 2.
Chapter: [0.051] Introduction to Trigonometry
If one zero of the polynomial p(x) = 6x2 + 37x – (k – 2) is reciprocal of the other, then find the value of k.
Chapter: [0.021] Polynomials
Find the sum and product of the roots of the quadratic equation 2x2 – 9x + 4 = 0.
Chapter: [0.021] Polynomials
Find the discriminant of the quadratic equation 4x2 – 5 = 0 and hence comment on the nature of roots of the equation.
Chapter: [0.023] Quadratic Equations
If a fair coin is tossed twice, find the probability of getting 'almost one head'.
Chapter: [0.07200000000000001] Probability
Evaluate:
`(5 cos^2 60^circ + 4 sec^2 30^circ - tan^2 45^circ)/(sin^2 30^circ + cos^2 30^circ)`
Chapter: [0.051] Introduction to Trigonometry
If A and B are acute angles such that sin (A – B) = 0 and 2 cos (A + B) – 1 = 0, then find angles A and B.
Chapter: [0.051] Introduction to Trigonometry
How many terms are there in an A.P. whose first and fifth terms are – 14 and 2, respectively and the last term is 62?
Chapter: [0.024] Arithmetic Progressions
Which term of the A.P. : 65, 61, 57, 53, .............. is the first negative term?
Chapter: [0.024] Arithmetic Progressions
Advertisements
Prove that `sqrt(5)` is an irrational number.
Chapter: [0.011000000000000001] Real Numbers
Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segments joining the points of contact to the centre.
Chapter: [0.042] Circles
The sum of first 15 terms of an A.P. is 750 and its first term is 15. Find its 20th term.
Chapter: [0.024] Arithmetic Progressions
Rohan repays his total loan of ₹ 1,18,000 by paying every month starting with the first installment of ₹ 1,000. If he increases the installment by ₹ 100 every month, what amount will be paid by him in the 30th installment? What amount of loan has he paid after 30th installment?
Chapter: [0.024] Arithmetic Progressions
Prove that `sqrt3` is an irrational number.
Chapter: [0.011000000000000001] Real Numbers
Find the value of 'p' for which the quadratic equation px(x – 2) + 6 = 0 has two equal real roots.
Chapter: [0.023] Quadratic Equations
From a soild cylinder of height 20 cm and diameter 12 cm, a conical cavity of height 8 cm and radius 6 cm is hallowed out. Find the total surface area of the remaining solid.
Chapter: [0.062] Surface Areas and Volumes
D is a point on the side BC of ∆ABC such that ∠ADC = ∠BAC. Prove that` \frac{"CA"}{"CD"}=\frac{"CB"}{"CA"} or "CA"^2 = "CB" × "CD".`
Chapter: [0.040999999999999995] Triangles [0.040999999999999995] Triangles [0.040999999999999995] Triangles
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR, prove that `("AB")/("PQ") = ("AD")/("PM")`.
Chapter: [0.040999999999999995] Triangles
A student was asked to make a model shaped like a cylinder with two cones attached to its ends by using a thin aluminium sheet. The diameter of the model is 3 cm and its total length is 12 cm. If each cone has a height of 2 cm, find the volume of air contained in the model.
Chapter: [0.062] Surface Areas and Volumes
In the given figure, ∠ADC = ∠BCA; prove that ΔACB ∼ ΔADC. Hence find BD if AC = 8 cm and AD = 3 cm.
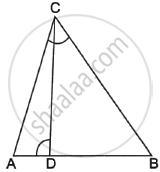
Chapter: [0.040999999999999995] Triangles
Prove that, if a line is drawn parallel to one side of a triangle to intersect the other two sides, then the two sides are divided in the same ratio.
Chapter: [0.040999999999999995] Triangles [0.040999999999999995] Triangles [0.040999999999999995] Triangles
Read the following passage:
Two schools 'P' and 'Q' decided to award prizes to their students for two games of Hockey ₹ x per student and Cricket ₹ y per student. School 'P' decided to award a total of ₹ 9,500 for the two games to 5 and 4 Students respectively; while school 'Q' decided to award ₹ 7,370 for the two games to 4 and 3 students respectively. |
Based on the above information, answer the following questions:
- Represent the following information algebraically (in terms of x and y).
- (a) What is the prize amount for hockey?
OR
(b) Prize amount on which game is more and by how much? - What will be the total prize amount if there are 2 students each from two games?
Chapter: [0.022000000000000002] Pair of Linear Equations in Two Variables
Read the following passage:
Jagdish has a field which is in the shape of a right angled triangle AQC. He wants to leave a space in the form of a square PQRS inside the field for growing wheat and the remaining for growing vegetables (as shown in the figure). In the field, there is a pole marked as O. |
Based on the above information, answer the following questions :
- Taking O as origin, coordinates of P are (–200, 0) and of Q are (200, 0). PQRS being a square, what are the coordinates of R and S?
- (a) What is the area of square PQRS?
OR
(b) What is the length of diagonal PR in square PQRS? - If S divides CA in the ratio K : 1, what is the value of K, where point A is (200, 800)?
Chapter: [0.031] Lines (In Two-dimensions)
Read the following passage:
Governing council of a local public development authority of Dehradun decided to build an adventurous playground on the top of a hill, which will have adequate space for parking.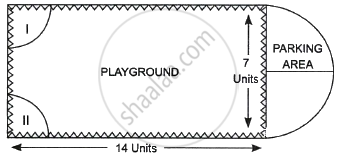 After survey, it was decided to build rectangular playground, with a semi-circular area allotted for parking at one end of the playground. The length and breadth of the rectangular playground are 14 units and 7 units, respectively. There are two quadrants of radius 2 units on one side for special seats. |
Based on the above information, answer the following questions:
- What is the total perimeter of the parking area?
- (a) What is the total area of parking and the two quadrants?
OR
(b) What is the ratio of area of playground to the area of parking area? - Find the cost of fencing the playground and parking area at the rate of ₹ 2 per unit.
Chapter: [0.061] Areas Related to Circles
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
CBSE previous year question papers Class 10 Mathematics with solutions 2022 - 2023
Previous year Question paper for CBSE Class 10 Maths-2023 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Mathematics, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of CBSE Class 10.
How CBSE Class 10 Question Paper solutions Help Students ?
• Question paper solutions for Mathematics will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
