Advertisements
Advertisements
Question
If cosB = `(1)/(3)` and ∠C = 90°, find sin A, and B and cot A.
Solution
cosB = `"Base"/"Hypotenuse" = "BC"/"AB"`
(AB)2 = (AC)2 + (BC)2
⇒ AC = `sqrt(("AB")^2 - ("BC")^2`
⇒ AC = `sqrt(3^2 - 1)`
= `sqrt(9 - 1)`
= `2sqrt(2)`
sin A = `"BC"/"AB" = "Perpendicular"/"Hypotenuse" = (1)/(3)`
tan B = `"AC"/"BC" = "Perpendicular"/"Base" = 2sqrt(2)`
cot A = `(1)/"tan A" = "BAse"/"Perpendicular" = "AC"/"BC" = 2sqrt(2)`.
APPEARS IN
RELATED QUESTIONS
If sin θ ,` sqrt (3)/2` find the value of all T- ratios of θ .
In a ΔABC , ∠B = 90° , AB = 12 cm and BC = 5 cm Find
(i) cos A (ii) cosec A (iii) cos C (iv) cosec C
Evaluate:
`4/(cot^2 30^0) +1/(sin^2 30^0) -2 cos^2 45^0 - sin^2 0^0`
If A and B are acute angles such that tan A =`1/3, tan B = 1/2 and tan (A + B) =` show that `A+B = 45^0`
If sin (A + B) = 1 and cos (A – B) = 1, 00 ≤ (A + B) ≤ 900 and A > B, then find A and B.
sin20° = cos ______°
Given: cos A = 0.6; find all other trigonometrical ratios for angle A.
Using the measurements given in the following figure:
(i) Find the value of sin θ and tan θ.
(ii) Write an expression for AD in terms of θ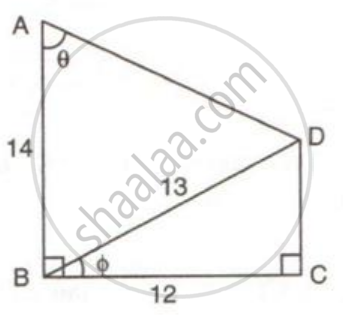
If cosec A + sin A = 5`(1)/(5)`, find the value of cosec2A + sin2A.
From the given figure, find the values of tan C
