Advertisements
Advertisements
Question
From the given figure, find the values of tan C
Solution
In the right ΔABD,
AD2 = AB2 – BD2
= 132 – 52
= 169 – 25
= 144
AD = `sqrt(144)`
= 12
In the right ΔADC,
AC2 = AD2 + DC2
= 122 + 162
= 144 + 256
= 400
AC = `sqrt(400)`
= 20
tan C = `"opposite side"/"adjacent side" = "AD"/"DC" = 12/16 = 3/4`
APPEARS IN
RELATED QUESTIONS
If A = B = 60°. Verify `tan (A - B) = (tan A - tan B)/(1 + tan tan B)`
In right angled triangle ABC. ∠C = 90°, ∠B = 60°. AB = 15 units. Find remaining angles and sides.
If sin θ = ` (a^2 - b^2)/(a^2+b^2)`find all the values of all T-ratios of θ .
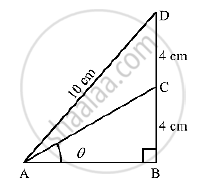
In the adjoining figure, `∠B = 90° , ∠BAC = theta° , BC = CD = 4cm and AD = 10 cm`. find (i) sin theta and (ii) `costheta`
Evaluate:
sin600 cos300 + cos600 sin300
Evaluate:
`(sin30°)/(cos 45°)+(cot45°)/(sec60° )- (sin60°)/(tan45°)+(cos30°)/(sin90°)`
From the following figure, find the values of :
(i) sin A
(ii) sec A
(iii) cos2 A + sin2A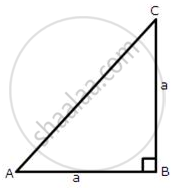
Given: sec A = `( 29 )/(21), "evaluate : sin A" - 1/tan "A"`
If sec A = `sqrt2`, find the value of :
`(3cos^2"A"+5tan^2"A")/(4tan^4"A"–sin^2"A")`
From the given figure, prove that θ + ∅ = 90°. Also prove that there are two other right angled triangles. Find sin α, cos β and tan ∅
