Advertisements
Advertisements
Question
If sec A = `sqrt2`, find the value of :
`(3cos^2"A"+5tan^2"A")/(4tan^4"A"–sin^2"A")`
Solution
Consider the diagram below :
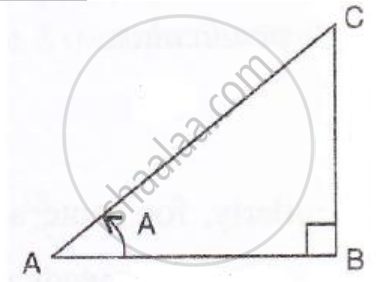
sec A =`sqrt2`
i.e.`"hypotenuse"/"base" =(sqrt2)/(1) ⇒ "AC"/"AB" =(sqrt2)/(1)`
Therefore if length of AB = x, length of AC =`sqrt2`
Since
AB2 + BC2 = AC2 ...[ Using Pythagoras Theorem]
(x)2 + BC2 = (`sqrt2"x")^2`
BC2 = 2x2 – x2 = x2
∴ BC = x ...(perpendicular)
Now
tan A = `"perpendicular"/"base" = ("x")/("x") =1`
sin A = `"perpendicular"/"hypotenuse" = ("x")/(sqrt2"x") = (1)/(sqrt2)`
cos A = `"base"/"hypotenuse" = ("x")/(sqrt2"x") = (1)/(sqrt2)`
Therefore
`(3cos^2"A"+5tan^2"A")/(4tan^4"A"–sin^2"A")`
= `(3(1/sqrt2)^2+5(1)^2)/(4(1)^2 – (1/sqrt2)^2)`
= `(13/2)/(7/2)`
= `(13)/(7)`
=`1(6)/(7)`
APPEARS IN
RELATED QUESTIONS
If sin 3θ = cos (θ – 6°) where 3θ and θ − 6° are acute angles, find the value of θ.
If sin θ = ` (a^2 - b^2)/(a^2+b^2)`find all the values of all T-ratios of θ .
In the figure of ΔPQR , ∠P = θ° and ∠R =∅° find
(i) `sqrt(X +1) cot ∅`
(ii)`sqrt( x^3 + x ^2) tantheta`
(iii) cos θ

If A = 300 , verify that:
(ii) cos 2A = `(1- tan^2A)/(1+tan^2A)`
Using the formula, cos A = `sqrt((1+cos2A)/2) ,`find the value of cos 300, it being given that cos 600 = `1/2`.
From the following figure, find the values of
(i) sin B
(ii) tan C
(iii) sec2 B - tan2B
(iv) sin2C + cos2C
In the diagram, given below, triangle ABC is right-angled at B and BD is perpendicular to AC.
Find:
(i) cos ∠DBC
(ii) cot ∠DBA

In triangle ABC, AD is perpendicular to BC. sin B = 0.8, BD = 9 cm and tan C = 1.
Find the length of AB, AD, AC, and DC.
If sin θ = `(8)/(17)`, find the other five trigonometric ratios.
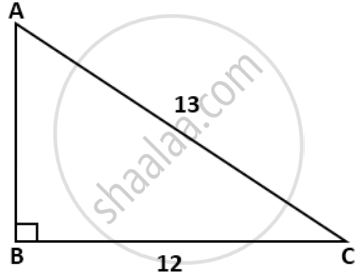
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: sin A cos A