Advertisements
Advertisements
Question
If sin 3θ = cos (θ – 6°) where 3θ and θ − 6° are acute angles, find the value of θ.
Solution
3θ, θ – 6 are an acute angle
We know that sin (90 – θ) = cos θ
sin 3θ = sin (90 – (θ - 6°))
sin 3θ = sin(90 – θ + 6°)
sin 3θ = sin (96° - θ)
3θ = 96° – θ
4θ = 96°
`θ = 96^@/4`
`θ = 24^@`
APPEARS IN
RELATED QUESTIONS
If 𝜃 = 30° verify `cos 2 theta = (1 - tan^2 theta)/(1 + tan^2 theta)`
If Sec 4A = cosec (A – 20°) where 4A is an acute angle, find the value of A.
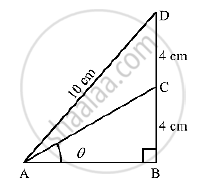
In the adjoining figure, `∠B = 90° , ∠BAC = theta° , BC = CD = 4cm and AD = 10 cm`. find (i) sin theta and (ii) `costheta`
Verify each of the following:
(i)`sin 60^0 cos 30^0-cos 60^0 sin 30^0`
If sin (A + B) = 1 and cos (A – B) = 1, 00 ≤ (A + B) ≤ 900 and A > B, then find A and B.
If tan x = `1(1)/(3)`, find the value of : 4 sin2x - 3 cos2x + 2
Using the measurements given in the following figure:
(i) Find the value of sin θ and tan θ.
(ii) Write an expression for AD in terms of θ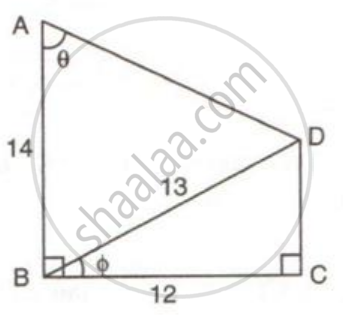
In ΔABC, ∠A = 90°. If AB = 5 units and AC = 12 units, find: sinB
If sin A = `(7)/(25)`, find the value of : `"cos A" + (1)/"cot A"`
If cosec θ = `(29)/(20)`, find the value of: `("sec" θ)/("tan" θ - "cosec" θ)`
