Advertisements
Advertisements
Question
In ΔABC, ∠A = 90°. If AB = 5 units and AC = 12 units, find: sinB
Solution
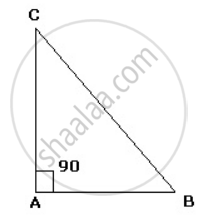
In ΔABC,
BC2 = AB2 + AC2
⇒ BC = `sqrt("AB"^2 + "AC"^2)`
⇒ BC = `sqrt(5^2 + 12^2)`
= `sqrt(169)`
= 13
AC = 12 units
BC = 13units
AB = 5units
Sin B
= `"Perpndicular"/"Hypoenuse"`
= `"AC"/"BC"`
= `(12)/(13)`
and
cos B
= `"Base"/"Hypotenuse"`
= `"AB"/"BC"`
= `(5)/(13)`.
APPEARS IN
RELATED QUESTIONS
if `cos theta = 5/13` find the value of `(sin^2 theta - cos^2 theta)/(2 sin theta cos theta) = 3/5`
if `sin theta = 3/5 " evaluate " (cos theta - 1/(tan theta))/(2 cot theta)`
If 𝜃 = 30° verify `cos 2 theta = (1 - tan^2 theta)/(1 + tan^2 theta)`
If 3 cot θ 4 , show that`((1-tan^2theta))/((1+tan^2theta)) = (cos^2theta - sin^2theta)`
Using the formula, tan 2A =`(2 tan A )/(1- tan^2 A)` find the value of tan 600, it being given that tan 300 = `1/sqrt(3)`.
Prove that
cosec (65 °+ θ) sec (25° − θ) − tan (55° − θ) + cot (35° + θ) = 0
If cot θ= 1; find the value of: 5 tan2 θ+ 2 sin2 θ- 3
Use the information given in the following figure to evaluate:
`(10)/sin x + (6)/sin y – 6 cot y`.
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: sin P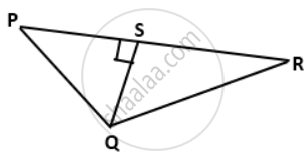
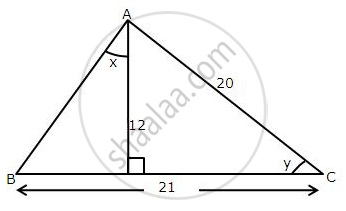
From the given figure, find the values of sec B