Advertisements
Advertisements
Question
If cot θ= 1; find the value of: 5 tan2 θ+ 2 sin2 θ- 3
Solution
Consider the diagram below :

cot θ = 1
i.e.`"base"/"perpendicular" = (1)/(1)`
Therefore if length of base = x, length of perpendicular = x
Since
base2 + perpendicular2 = hypotenuse2 ...[ Using Pythagooras Theorem]
(x)2 + (x)2 = hypotenuse2
hypotenuse2 = x2 + x2 = 2x
∴ hypotenuse =`sqrt2x`
Now
sin θ = `"perpendicular"/"hypotenuse" = (x)/(sqrt2x) = (1)/(sqrt2)`
tan θ = `"perpendicular"/"base" = (x)/(x) = 1`
Therefore
5tan2 θ + 2sin2 θ – 3
= `5 (1)^2 + 2 (1/sqrt2)^2 – 3`
= 5 + 1 – 3
=3
APPEARS IN
RELATED QUESTIONS
In right angled triangle ΔABC at B, ∠A = ∠C. Find the values of sin A sin B + cos A cos B
If cos 2θ = sin 4θ where 2θ, 4θ are acute angles, find the value of θ.
If tan θ = `1/sqrt(7) `show that ` (cosec ^2 θ - sec^2 θ)/(cosec^2 θ + sec^2 θ ) = 3/4`
If tan `theta = a/b`, show that `((a sin theta - b cos theta))/((a sin theta + bcos theta))= ((a^2-b^2))/(a^2+b^2)`
Using the formula, cos A = `sqrt((1+cos2A)/2) ,`find the value of cos 300, it being given that cos 600 = `1/2`.
cos 40° = sin ______°
In the given figure;
BC = 15 cm and sin B = `(4)/(5)`
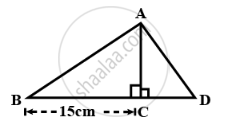
- Calculate the measure of AB and AC.
- Now, if tan ∠ADC = 1; calculate the measures of CD and AD.
Also, show that: tan2B - `1/cos^2 "B" = – 1 .`
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: cos C
If sin A = `(7)/(25)`, find the value of : `(2"tanA")/"cot A - sin A"`
If cosec θ = `(29)/(20)`, find the value of: `("sec" θ)/("tan" θ - "cosec" θ)`
