Advertisements
Advertisements
प्रश्न
If cot θ= 1; find the value of: 5 tan2 θ+ 2 sin2 θ- 3
उत्तर
Consider the diagram below :

cot θ = 1
i.e.`"base"/"perpendicular" = (1)/(1)`
Therefore if length of base = x, length of perpendicular = x
Since
base2 + perpendicular2 = hypotenuse2 ...[ Using Pythagooras Theorem]
(x)2 + (x)2 = hypotenuse2
hypotenuse2 = x2 + x2 = 2x
∴ hypotenuse =`sqrt2x`
Now
sin θ = `"perpendicular"/"hypotenuse" = (x)/(sqrt2x) = (1)/(sqrt2)`
tan θ = `"perpendicular"/"base" = (x)/(x) = 1`
Therefore
5tan2 θ + 2sin2 θ – 3
= `5 (1)^2 + 2 (1/sqrt2)^2 – 3`
= 5 + 1 – 3
=3
APPEARS IN
संबंधित प्रश्न
If cos 2θ = sin 4θ where 2θ, 4θ are acute angles, find the value of θ.
If sin θ = ` (a^2 - b^2)/(a^2+b^2)`find all the values of all T-ratios of θ .
If sin ∝ = `1/2` prove that (3cos∝ - `4cos^2` ∝)=0
If sin (A + B) = 1 and cos (A – B) = 1, 00 ≤ (A + B) ≤ 900 and A > B, then find A and B.
If sin (A – B) = `1/2` and cos (A + B) = `1/2, 0^0 ≤ (A + B) ≤ 90^0` and A > B, then find A and B.
cos 40° = sin ______°
Given: 4 cot A = 3
find :
(i) sin A
(ii) sec A
(iii) cosec2A - cot2A.
In a right-angled triangle, it is given that A is an acute angle and tan A = `(5) /(12)`.
find the value of :
(i) cos A
(ii) sin A
(iii) ` (cosA+sinA)/(cosA– sin A)`
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`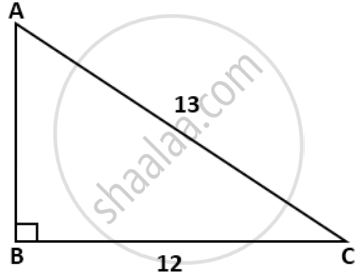
From the given figure, find all the trigonometric ratios of angle B
