Advertisements
Advertisements
प्रश्न
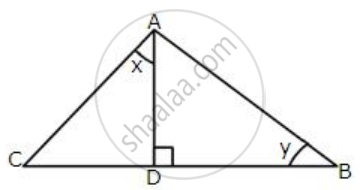
In the following figure:
AD ⊥ BC, AC = 26 CD = 10, BC = 42, ∠DAC = x and ∠B = y.
Find the value of :
(i) cot x
(ii) `1/sin^2 y – 1/tan^2 y`
(iii) `6/cos x – 5/cos y + 8 tan y`.
उत्तर
Given the angle, DAC = 90° and ∠ADB = 90° in the figure
⇒ AC2 = AD2 + DC2 ...(AC is hypotenuse in ΔADC)
⇒ AD2 = AC2 - DC2
⇒ AD2 = 262 – 102
∴ AD2 = 576
∴ AD2 = `sqrt(576)`
∴ AD = 24
Again, In ΔABD
⇒ AB2 = AD2 + BD2 ...(AB is hypotenuse in ΔABD)
⇒ AB2 = 242 + 322
⇒ AB2 = 576 + 1024
⇒ AB2 = `sqrt1600`
∴ AB = 40
Now
(i) cot x = `"base"/"perpendicular" = "AD"/"CD" = (24)/(10) = 2. 4`
(ii) sin y = `"perpendicular"/"hypotenuse" ="AD"/"AB" = (24)/(40) = (3)/(5)`
tan y = `"perpendicular"/"base" ="AD"/"BD" = (24)/(32) = (3)/(4)`
Therefore
`1/sin^2 y – 1/tan^2 y`
= `1/(3/5)^2 – 1/(3/4)^2`
= `(5/3)^2 - (4/3)^2`
= `(25)/(9) – (16)/(9)`
= `(9)/(9)`
= 1
(iii) tan y =`"perpendicular"/"base" = "AD"/"BD" = (24)/(32) = (3)/(4)`
cos x = `"base"/"hypotenuse" = "AD"/"AC" = (24)/(26) = (12)/(13)`
cos y = `"base"/"hypotenuse" = "BD"/"AB" = (32)/(40) = (4)/(5)`
Therefore
`6/ cos x – 5/ cos y + 8tan y`
= `6/(12/13) – 5/(4/5) + 8(3/4)`
= `(13)/(2) – (25)/(4) + 6`
= `(26 – 25 + 24)/(4)`
= `(25)/(4)`
= `6(1)/(4)`
APPEARS IN
संबंधित प्रश्न
If A = B = 60°, verify that cos (A − B) = cos A cos B + sin A sin B
If Sin (A + B) = 1 and cos (A – B) = 1, 0° < A + B ≤ 90° A ≥ B. Find A & B
In the figure of ΔPQR , ∠P = θ° and ∠R =∅° find
(i) `sqrt(X +1) cot ∅`
(ii)`sqrt( x^3 + x ^2) tantheta`
(iii) cos θ

If A = 450, verify that :
(i) sin 2A = 2 sin A cos A
From the following figure, find the values of:
- sin A
- cos A
- cot A
- sec C
- cosec C
- tan C

Form the following figure, find the values of:
- cos B
- tan C
- sin2B + cos2B
- sin B. cos C + cos B. sin C

From the following figure, find the values of :
(i) sin A
(ii) sec A
(iii) cos2 A + sin2A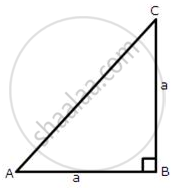
If tan = 0.75, find the other trigonometric ratios for A.
If sin θ = `"a"/sqrt("a"^2 + "b"^2)`, then show that b sin θ = a cos θ
Statement A (Assertion): For 0 < θ ≤ 90°, cosec θ – cot θ and cosec θ + cot θ are reciprocal of each other.
Statement R (Reason): cosec2 θ – cot2 θ = 1
