Advertisements
Advertisements
प्रश्न
From the following figure, find the values of:
- sin A
- cos A
- cot A
- sec C
- cosec C
- tan C

उत्तर
Given angle ABC = 90°
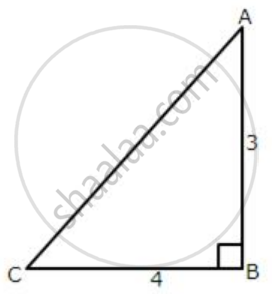
⇒ AC2 = AB2 + BC2 ...(AC is hypotenuse)
⇒ AC2 = 32 + 42
∴ AC2 = 9 + 16
∴ AC2 = 25
∴ AC2 = `sqrt25`
∴ AC = 5
(i) sin A = `" perpendicular"/" hypotenuse" = "BC"/ "AC" = 4/5`
(ii) cos A = `"base"/"hypotenuse" = "AB"/ "AC" = 3/5`
(iii) cot A = `"base "/"perpendicular" = "AB"/ "BC" = 3/4`
(iv) sec C = `"hypotenuse "/"base" = "AC"/ "BC" = 5/4`
(v) cosec C = `"hypotenuse"/" perpendicular" = "AC"/ "AB" = 5/3`
(vi) tan C = `" perpendicular"/"base" = "AB"/ "BC" = 3/4`
APPEARS IN
संबंधित प्रश्न
If sin θ = cos (θ – 45°), where θ – 45° are acute angles, find the degree measure of θ
If A, B, C are the interior angles of a ΔABC, show that `cos[(B+C)/2] = sin A/2`
If 2θ + 45° and 30° − θ are acute angles, find the degree measure of θ satisfying Sin (20 + 45°) = cos (30 - θ°)
If ∠A and ∠B are acute angles such that tan A= Tan B then prove that ∠A = ∠B
Given: tan A = `4/3 , "find" : ("cosec""A")/(cot "A"– sec "A")`
In a right-angled triangle, it is given that A is an acute angle and tan A = `(5) /(12)`.
find the value of :
(i) cos A
(ii) sin A
(iii) ` (cosA+sinA)/(cosA– sin A)`
In the given figure, triangle ABC is right-angled at B. D is the foot of the perpendicular from B to AC. Given that BC = 3 cm and AB = 4 cm.
find :
- tan ∠DBC
- sin ∠DBA

Given : 17 cos θ = 15;
Find the value of: tan θ + 2 secθ .
If 3 cos A = 4 sin A, find the value of :
(i) cos A(ii) 3 - cot2 A + cosec2A.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
sinB = `sqrt(3)/(2)`
