Question

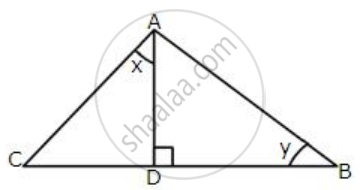
In the following figure: 

AD ⊥ BC, AC = 26 CD = 10, BC = 42, ∠DAC = x and ∠B = y.

Find the value of :

(i) cot x

(ii) `1/sin^2 y – 1/tan^2 y`

(iii) `6/cos x – 5/cos y + 8 tan y`.

Answer 1

Given the angle, DAC = 90°  and ∠ADB = 90° in the figure

⇒ AC² = AD² + DC²  ...(AC is hypotenuse in ΔADC)

⇒ AD² = AC² - DC²

⇒ AD² = 26² – 10²

∴ AD² = 576

∴ AD² = `sqrt(576)`

∴ AD = 24

Again, In ΔABD

⇒ AB² = AD² + BD²   ...(AB is hypotenuse in ΔABD)

⇒ AB² = 24² + 32²

⇒ AB² = 576 + 1024

⇒ AB² = `sqrt1600`

∴ AB = 40

Now
(i) cot x = `"base"/"perpendicular" = "AD"/"CD" = (24)/(10) = 2. 4`

(ii) sin y = `"perpendicular"/"hypotenuse" ="AD"/"AB" = (24)/(40) = (3)/(5)`

tan y = `"perpendicular"/"base" ="AD"/"BD" = (24)/(32) = (3)/(4)`

Therefore

`1/sin^2 y – 1/tan^2 y`

= `1/(3/5)^2 – 1/(3/4)^2`

= `(5/3)^2 - (4/3)^2`

= `(25)/(9)  –  (16)/(9)`

= `(9)/(9)`

= 1

(iii) tan y =`"perpendicular"/"base" = "AD"/"BD" = (24)/(32) = (3)/(4)`

cos x = `"base"/"hypotenuse" = "AD"/"AC" = (24)/(26) = (12)/(13)`

cos y = `"base"/"hypotenuse" = "BD"/"AB" = (32)/(40) = (4)/(5)`  

Therefore
`6/ cos x – 5/ cos y + 8tan y`

= `6/(12/13) – 5/(4/5) + 8(3/4)`

= `(13)/(2) – (25)/(4) + 6`

= `(26  –  25 + 24)/(4)`

= `(25)/(4)`

= `6(1)/(4)`