Advertisements
Advertisements
प्रश्न
From the following figure, find:
(i) y
(ii) sin x°
(iii) (sec x° - tan x°) (sec x° + tan x°)

उत्तर
Consider the given figure :

(i) Since the triangle is a right-angled triangle, so using Pythagorean Theorem
22 = y2 + 12
y2 = 4 – 1 = 3
y =
(ii) sin x° =
(iii) tan x° =
sec x° =
Therefore
( sec x° – tan x°) ( sec x° + tan x°)
= (2–
= 4 – 3
= 1
APPEARS IN
संबंधित प्रश्न
If 2θ + 45° and 30° − θ are acute angles, find the degree measure of θ satisfying Sin (20 + 45°) = cos (30 - θ°)
If tan
If sin ∝ =
Evaluate:
In the adjoining figure, ΔABC is right-angled at B and ∠A = 450. If AC = 3
In triangle ABC; ∠ABC = 90°, ∠CAB = x°, tan x° =
In a right-angled triangle PQR, ∠PQR = 90°, QS ⊥ PR and tan R =
From the given figure, find the values of sin B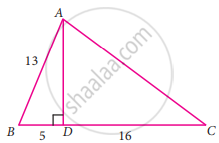
If cos θ : sin θ = 1 : 2, then find the value of
Given that sin α =
