Advertisements
Advertisements
Question
If cosec θ = `(29)/(20)`, find the value of: `("sec" θ)/("tan" θ - "cosec" θ)`
Solution

Consider ΔABC, where ∠A = 90°
⇒ cosec θ = `"Hypotenuse"/"Perpendicular" = "BC"/"AB" = (29)/(20)`
By Pythagoras theorem,
BC2 = AB2 + AC2
⇒ AC2 = BC2 - AB2
= 292 - 202
= 841 - 400
= 441
⇒ AC = 21
Now,
sec θ = `"Hypotenuse"/"Base" = "BC"/"AC" = (29)/(21)`
tan θ = `"Perpendicular"/"Base" = "AB"/"AC" = (20)/(21)`
⇒ cot θ = `(1)/"tan θ " = (21)/(20)`
`"sec θ"/("tan" θ - "cosec" θ")`
= `(29/21)/(20/21 - 29/20)`
= `(29/21)/(-209/420)`
= `(29)/(21) xx (-420)/(209)`
= `(-580)/(209)`.
APPEARS IN
RELATED QUESTIONS
If Sec 4A = cosec (A – 20°) where 4A is an acute angle, find the value of A.
Evaluate:
sin600 cos300 + cos600 sin300
If A = 600 and B = 300, verify that:
(iii) tan (A-B) = `(tan A-tanB)/(1+tan A tan B)`
tan 30° × tan ______° = 1
Prove that
sin (50° + θ ) − cos (40° − θ) + tan 1° tan 10° tan 80° tan 89° = 1.
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: cot C
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of sin x
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of tan x. cot y
From the given figure, find the values of sin B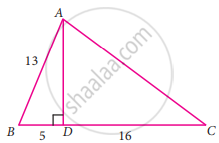
Evaluate: `5/(cot^2 30^circ) + 1/(sin^2 60^circ) - cot^2 45^circ + 2 sin^2 90^circ`.
