Advertisements
Advertisements
Question
From the given figure, find the values of cosec C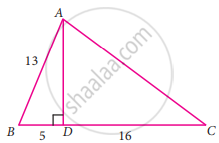
Solution
In the right ΔABD,
AD2 = AB2 – BD2
= 132 – 52
= 169 – 25
= 144
AD = `sqrt(144)`
= 12
In the right ΔADC,
AC2 = AD2 + DC2
= 122 + 162
= 144 + 256
= 400
AC = `sqrt(400)`
= 20
cosec C = `"hypotenuse"/"opposite side" = "AC"/"AD" = 20/12 = 5/3`
APPEARS IN
RELATED QUESTIONS
if `sin theta = 3/5 " evaluate " (cos theta - 1/(tan theta))/(2 cot theta)`
If 8 tan A = 15, find sin A – cos A.
If tan θ = `1/sqrt(7) `show that ` (cosec ^2 θ - sec^2 θ)/(cosec^2 θ + sec^2 θ ) = 3/4`
If sin θ = `3/4` show that `sqrt((cosec^2theta - cot^2theta)/(sec^2theta-1)) =sqrt(7)/3`
If 3 cot θ 4 , show that`((1-tan^2theta))/((1+tan^2theta)) = (cos^2theta - sin^2theta)`
Evaluate:
sin600 cos300 + cos600 sin300
Evaluate:
cos600 cos300− sin600 sin300
If A = 300 , verify that:
(ii) cos 2A = `(1- tan^2A)/(1+tan^2A)`
In triangle ABC, AB = AC = 15 cm and BC = 18 cm. Find:
- cos B
- sin C
- tan2 B - sec2 B + 2
If cosB = `(1)/(3)` and ∠C = 90°, find sin A, and B and cot A.
