Advertisements
Advertisements
प्रश्न
From the given figure, find the values of cosec C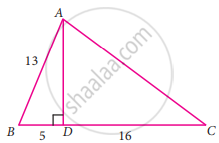
उत्तर
In the right ΔABD,
AD2 = AB2 – BD2
= 132 – 52
= 169 – 25
= 144
AD = `sqrt(144)`
= 12
In the right ΔADC,
AC2 = AD2 + DC2
= 122 + 162
= 144 + 256
= 400
AC = `sqrt(400)`
= 20
cosec C = `"hypotenuse"/"opposite side" = "AC"/"AD" = 20/12 = 5/3`
APPEARS IN
संबंधित प्रश्न
If 2θ + 45° and 30° − θ are acute angles, find the degree measure of θ satisfying Sin (20 + 45°) = cos (30 - θ°)
If sin ∝ = `1/2` prove that (3cos∝ - `4cos^2` ∝)=0
If A = 600 and B = 300, verify that:
(ii) cos (A – B) = cos A cos B + sin A sin B
Using the formula, sin A = `sqrt((1-cos 2A)/2) ` find the value of sin 300, it being given that cos 600 = `1/2`
cos 40° = sin ______°
In the given figure;
BC = 15 cm and sin B = `(4)/(5)`
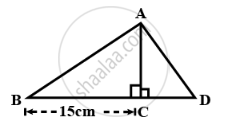
- Calculate the measure of AB and AC.
- Now, if tan ∠ADC = 1; calculate the measures of CD and AD.
Also, show that: tan2B - `1/cos^2 "B" = – 1 .`
If sin A + cosec A = 2;
Find the value of sin2 A + cosec2 A.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
sinB = `sqrt(3)/(2)`
In ΔABC, ∠A = 90°. If AB = 5 units and AC = 12 units, find: sinB
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of sin x
