Advertisements
Advertisements
Question
From the following figure, find the values of :
(i) sin A
(ii) sec A
(iii) cos2 A + sin2A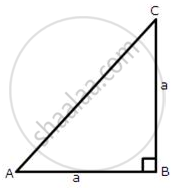
Solution
Given angle, ABC = 90° in the figure
⇒ AC2 = AB2 + BC2 ...(AC is hypotenuse in Δ ABC )
⇒ AC2 = a2 + a2
∴ AC2 = 2a2 and AC =`sqrt2a`
Now
(i) sin A = `"perpendicular"/"hypotenuse" = "BC"/"AC" = a/(sqrt2a) = 1/(sqrt2)`
(ii) sec A = `"hypotenuse"/"base" = "AC"/"AB" = (sqrt2a)/a = sqrt2`
(iii) sin A = `"perpendicular"/"hypotenuse" = "BC"/"AC" = a/(sqrt2a) = 1/(sqrt2)`
cos A = `"base"/"hypotenuse" = "AB"/"AC" = a/(sqrt2a) = 1/(sqrt2)`
cos2A + sin2A = `(1/(sqrt2))^2 + (1 /(sqrt2))^2`
= `(1)/(2) +(1)/ (2)`
= 1
APPEARS IN
RELATED QUESTIONS
In rectangle ABCD AB = 20cm ∠BAC = 60° BC, calculate side BC and diagonals AC and BD.
If cos θ = `7/25` find the value of all T-ratios of θ .
Verify each of the following:
(iv) `2 sin 45^0 cos 45^0`
If sin (A – B) = `1/2` and cos (A + B) = `1/2, 0^0 ≤ (A + B) ≤ 90^0` and A > B, then find A and B.
Given: sin θ = `p/q`.
Find cos θ + sin θ in terms of p and q.
If cot θ= 1; find the value of: 5 tan2 θ+ 2 sin2 θ- 3
In triangle ABC, ∠B = 90° and tan A = 0.75. If AC = 30 cm, find the lengths of AB and BC.
If sin A = `(7)/(25)`, find the value of : `(2"tanA")/"cot A - sin A"`
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`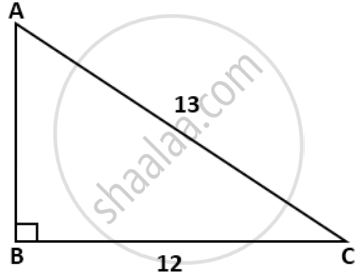
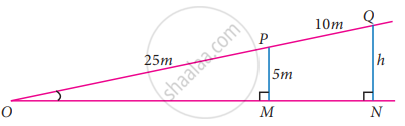
A boy standing at a point O finds his kite flying at a point P with distance OP = 25 m. It is at a height of 5 m from the ground. When the thread is extended by 10 m from P, it reaches a point Q. What will be the height QN of the kite from the ground? (use trigonometric ratios)