Advertisements
Advertisements
Question
Given 15 cot A = 8. Find sin A and sec A.
Solution
Consider a right-angled triangle, right-angled at B.
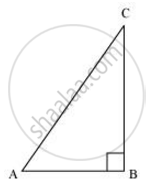
It is given that
cot A = `8/15`
`("AB")/("BC")=8/15`
Let AB be 8k.
Therefore, BC will be 15k, where k is a positive integer.
Applying Pythagoras theorem in ΔABC, we obtain
AC2 = AB2 + BC2
= (8k)2 + (15k)2
= 64k2 + 225k2
= 289k2
AC = 17k
sin A = `(15k)/(17k)`
= `15/17`
sec A = `("AC")/("AB")`
= `17/8`
RELATED QUESTIONS
if `cos theta = 5/13` find the value of `(sin^2 theta - cos^2 theta)/(2 sin theta cos theta) = 3/5`
f θ = 30°, verify that cos 3θ = 4 cos3 θ − 3 cos θ
If sin θ = ` (a^2 - b^2)/(a^2+b^2)`find all the values of all T-ratios of θ .
In a ΔABC , ∠B = 90° , AB = 12 cm and BC = 5 cm Find
(i) cos A (ii) cosec A (iii) cos C (iv) cosec C
If sin ∝ = `1/2` prove that (3cos∝ - `4cos^2` ∝)=0
In the figure of ΔPQR , ∠P = θ° and ∠R =∅° find
(i) `sqrt(X +1) cot ∅`
(ii)`sqrt( x^3 + x ^2) tantheta`
(iii) cos θ

If x = cot A + cos A and y = cot A – cos A then prove that `((x-y)/(x+y))^2 + ((x-y)/2)^2=1`
Evaluate:
sin600 cos300 + cos600 sin300
Evaluate:
`2cos^2 60^0+3 sin^2 45^0 - 3 sin^2 30^0 + 2 cos^2 90 ^0`
If A = 600 and B = 300, verify that:
(i) sin (A + B) = sin A cos B + cos A sin B
If A = 600 and B = 300, verify that:
(iii) tan (A-B) = `(tan A-tanB)/(1+tan A tan B)`
In the adjoining figure, ΔABC is a right-angled triangle in which ∠B = 900, ∠300 and AC = 20cm. Find (i) BC, (ii) AB.
In triangle ABC, AB = AC = 15 cm and BC = 18 cm, find cos ∠ABC.
In the given figure; ∠C = 90o and D is mid-point of AC.
Find :
(i) `(tan∠CAB)/ (tan∠CDB)` (ii) `(tan∠ABC)/ (tan∠DBC)`
If 5 cos = 6 sin ; evaluate:
(i) tan θ
(ii) `(12 sin θ – 3 cos θ)/(12 sin θ + 3 cos θ)`
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of tan x. cot y
In a right-angled triangle PQR, ∠PQR = 90°, QS ⊥ PR and tan R =`(5)/(12)`, find the value of tan ∠SQR
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: sin A cos A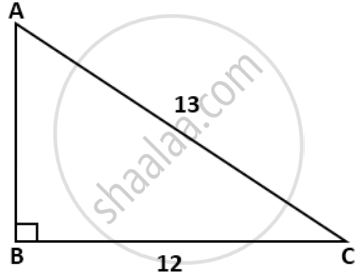
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`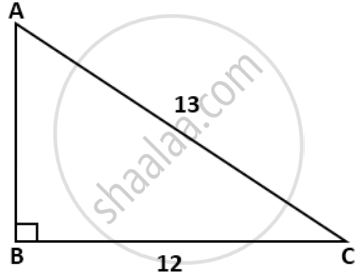
If cos A = `3/5`, then find the value of `(sin"A" - cos"A")/(2tan"A")`
