Advertisements
Advertisements
Question
In triangle ABC, AB = AC = 15 cm and BC = 18 cm, find cos ∠ABC.
Solution
Consider the figure below :
In the isosceles ΔABC, AB = AC = 15cm and BC =18cm the perpendicular drawn from angle A to the side BC divides the side BC into two equal parts BD = DC = 9 cm
cos ∠ABC = `"base"/"hypotenuse" = "BD"/"AB" = (9)/(15) = (3)/(5)`
APPEARS IN
RELATED QUESTIONS
If A = 30° and B = 60°, verify that cos (A + B) = cos A cos B − sin A sin B
If 3 cot θ 4 , show that`((1-tan^2theta))/((1+tan^2theta)) = (cos^2theta - sin^2theta)`
In a ΔABC , ∠B = 90° , AB= 24 cm and BC = 7 cm find (i) sin A (ii) cos A (iii) sin C (iv) cos C
Evaluate:
`2cos^2 60^0+3 sin^2 45^0 - 3 sin^2 30^0 + 2 cos^2 90 ^0`
Prove that
cosec (65 °+ θ) sec (25° − θ) − tan (55° − θ) + cot (35° + θ) = 0
If cosec θ = `sqrt5`, find the value of:
- 2 - sin2 θ - cos2 θ
- 2 + `1/sin^2"θ" – cos^2"θ"/sin^2"θ"`
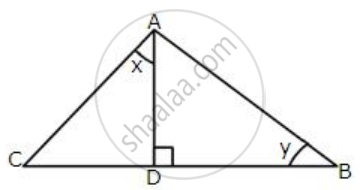
In the following figure:
AD ⊥ BC, AC = 26 CD = 10, BC = 42, ∠DAC = x and ∠B = y.
Find the value of :
(i) cot x
(ii) `1/sin^2 y – 1/tan^2 y`
(iii) `6/cos x – 5/cos y + 8 tan y`.
In ΔABC, ∠A = 90°. If AB = 5 units and AC = 12 units, find: sinB
In ΔABC, ∠A = 90°. If AB = 5 units and AC = 12 units, find: tan B.
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cosec C
