Advertisements
Advertisements
प्रश्न
Evaluate the following limits:
`lim_(alpha -> 0) (sin(alpha^"n"))/(sin alpha)^"m"`
उत्तर
We know `lim_(x -> 0) (sin x)/x` = 1
`lim_(alpha -> 0) (sin(alpha^"n"))/(sin alpha)^"m" = lim_(alpha -> 0) (sin (alpha^"n"))/(1/alpha^"n" (alpha^"n")) xx (alpha^(1/"m") * alpha^"m")/(sin alpha)^"m"`
= `lim_(alpha -> 0) alpha^"n" * (sin(alpha^"n"))/alpha^"n" xx 1/alpha^"m" * 1/(((sinalpha)^"m")/(alpha^"m"))`
= `lim_(alpha -> 0) alpha^("n" - "m") *(sin(alpha^"n"))/alpha^"n" xx 1/((sinalpha)/alpha)^"m"`
= `(lim_(alpha -> 0) alpha^("n" - "m")) xx (lim_(alpha^"n" -> 0) (sin(alpha^"n"))/alpha^"n") xx 1/(lim_(alpha -> 0) ((sin alpha)/alpha)^"m")`
Case (i) m = n
`lim_(alpha -> 0) (sin(alpha^"n"))/(sin alpha)^"m" = (lim_(alpha -> 0) alpha^("m" - "m")) (lim_(alpha^"m" -> 0) (sin(alpha^"m"))/alpha^"m") xx 1/(lim_(alpha -> 0) ((sin alpha)/alpha)^"m")`
= `(lim_(alpha -> 0) alpha^0) xx 1 xx 1/1`
`lim_(alpha -> 0) (sin(alpha^"n"))/(sin alpha)^"m" = 1 xx 1 xx 1` = 1
Case (ii) m > n then n – m < 0
`lim_(alpha -> 0) (sin(alpha^"n"))/(sin alpha)^"m" = (lim_(alpha -> 0) alpha^("n" - "m")) (lim_(apha^"m" -> 0) (sin(alpha^"m"))/alpha^"m") xx 1/((lim_(alpha-> 0) ((sin alpha)/alpha)^"m"))`
= `lim_(alpha -> 0) 1/(alpha^("m" - "n")) xx 1 xx 1/1`
= `oo xx 1 xx 1 = oo`
Since `lim_(alpha -> 0) 1/(alpha^("m" - "n")) = lim_(alpha -> 0) (1/0)^("m" - "n") = oo`
Case (iii) m < n then n – m > 0
`lim_(alpha -> 0) (sin(alpha^"n"))/(sin alpha)^"m" = (lim_(alpha -> 0) alpha^("n" - "m")) (lim_(alpha^"m" -> 0) (sin(alpha^"m"))/(alpha^"m")) xx 1/(lim_(alpha-> 0) ((sin alpha)/alpha)^"m"`
= `(0)^("n" - "m") xx 1 xx 1`
= 0
∴ `lim_(alpha -> 0) (sin(alpha^"n"))/(sin alpha)^"m" = {{:(1, "if", "m" = "n"),(oo, "if", "m" > "n"),(0, "if", m < n):}`
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit :
`lim_(x -> 0)[((1 - x)^8 - 1)/((1 - x)^2 - 1)]`
Evaluate the following limit :
`lim_(x -> 7) [(x^3 - 343)/(sqrt(x) - sqrt(7))]`
Evaluate the following limit :
`lim_(x -> 1) [(x + x^3 + x^5 + ... + x^(2"n" - 1) - "n")/(x - 1)]`
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 2) (x - 2)/(x^2 - 4)`
| x | 1.9 | 1.99 | 1.999 | 2.001 | 2.01 | 2.1 |
| f(x) | 0.25641 | 0.25062 | 0.250062 | 0.24993 | 0.24937 | 0.24390 |
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) (sqrt(x + 3) - sqrt(3))/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.001 | 0.01 | 0.1 |
| f(x) | 0.2911 | 0.2891 | 0.2886 | 0.2886 | 0.2885 | 0.28631 |
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> - 3) (sqrt(1 - x) - 2)/(x + 3)`
| x | – 3.1 | – 3.01 | – 3.00 | – 2.999 | – 2.99 | – 2.9 |
| f(x) | – 0.24845 | – 0.24984 | – 0.24998 | – 0.25001 | – 0.25015 | – 0.25158 |
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 5) |x - 5|/(x - 5)`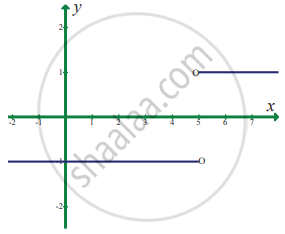
Evaluate : `lim_(x -> 3) (x^2 - 9)/(x - 3)` if it exists by finding `f(3^-)` and `f(3^+)`
Evaluate the following limits:
`lim_(x -> 0) (sqrt(x^2 + 1) - 1)/(sqrt(x^2 + 16) - 4)`
Find the left and right limits of f(x) = tan x at x = `pi/2`
Evaluate the following limits:
`lim_(x -> oo) (1 + x - 3x^3)/(1 + x^2 +3x^3)`
Evaluate the following limits:
`lim_(x -> 0) (sin^3(x/2))/x^2`
Evaluate the following limits:
`lim_(x -> oo) x [3^(1/x) + 1 - cos(1/x) - "e"^(1/x)]`
Evaluate the following limits:
`lim_(x -> 0) ("e"^("a"x) - "e"^("b"x))/x`
Evaluate the following limits:
`lim_(x -> 0) (tan x - sin x)/x^3`
Choose the correct alternative:
If `f(x) = x(- 1)^([1/x])`, x ≤ 0, then the value of `lim_(x -> 0) f(x)` is equal to
Choose the correct alternative:
`lim_(alpha - pi/4) (sin alpha - cos alpha)/(alpha - pi/4)` is
Choose the correct alternative:
`lim_(x -> oo) (1/"n"^2 + 2/"n"^2 + 3/"n"^2 + ... + "n"/"n"^2)` is
The value of `lim_(x→0)(sin(ℓn e^x))^2/((e^(tan^2x) - 1))` is ______.
