Advertisements
Advertisements
प्रश्न
Figure shows a transparent hemisphere of radius 3.0 cm made of a material of refractive index 2.0. (a) A narrow beam of parallel rays is incident on the hemisphere as shown in the figure. Are the rays totally reflected at the plane surface? (b) Find the image formed by the refraction at the first surface. (c) Find the image formed by the reflection or by the refraction at the plane surface. (d) Trace qualitatively the final rays as they come out of the hemisphere.

उत्तर
Given,
The radius of the transparent hemisphere (R) = 3.0 cm
Refractive index of the material (μ2) = 2.0
Let the critical angle be θc
∴ critical angle is given by θc = \[\sin^{- 1} \left( \frac{1}{\mu_2} \right) = \sin^{- 1} \left( \frac{1}{2} \right) = 30^\circ\]
(a)
From the figure it is seen that the angle of incidence is greater than the critical angle, so the rays are totally reflected at the plane surface.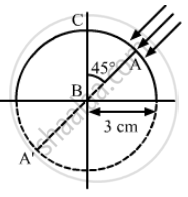
(b) Using the lens equation:
\[\frac{\mu_2}{v} - \frac{\mu_1}{u} = \frac{\mu_2 - \mu_1}{R}\]
\[ \Rightarrow \frac{2}{v} - \left( - \frac{1}{\infty} \right) = \frac{2 - 1}{3} (\text{ for parallel rays u = \infty })\]
\[ \Rightarrow \frac{2}{v} = \frac{1}{3}\]
\[ \Rightarrow v = 6 cm\]
If we complete the sphere then the image will be formed diametrically opposite to A.
(c)
By internal reflection, the image is formed in front of A.
APPEARS IN
संबंधित प्रश्न
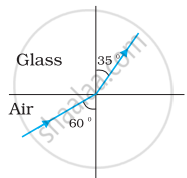
Figures (a) and (b) show the refraction of a ray in air incident at 60° with the normal to a glass-air and water-air interface, respectively. Predict the angle of refraction in the glass when the angle of incidence in water is 45° with the normal to a water-glass interface [Figure (c)].
 |
 |
 |
| (a) | (b) | (c) |
Light incident normally on a plane mirror attached to a galvanometer coil retraces backward as shown in Figure. A current in the coil produces a deflection of 3.5° of the mirror. What is the displacement of the reflected spot of light on a screen placed 1.5 m away?

Monochromatic light of wavelength 589 nm is incident from air on a water surface. If µ for water is 1.33, find the wavelength, frequency and speed of the refracted light.
Why does a diamond shine more than a glass piece cut to the same shape?
A vessel contains water up to a height of 20 cm and above it an oil up to another 20 cm. The refractive indices of the water and the oil are 1.33 and 1.30 respectively. Find the apparent depth of the vessel when viewed from above.
Choose the correct option.
There are different fish, monkeys, and water of the habitable planet of the star Proxima b. A fish swimming underwater feels that there is a monkey at 2.5 m on the top of a tree. The same monkey feels that the fish is 1.6 m below the water surface. Interestingly, height of the tree and the depth at which the fish is swimming are exactly same. Refractive index of that water must be
Stars twinkle due to ______.
Obtain the equation for critical angle.
Explain the reason for glittering of diamond.
What is mirage?
What is looming?
Write a short note on the prisms making use of total internal reflection.
Obtain the equation for radius of illumination (or) Snell’s window.
A light travels through water in the beaker. The height of water column is 'h'. Refractive index of water is 'μw'. If c is velocity of light in air, the time taken by light to travel through water will ______.
The critical angle is maximum when light travels from ______.
`(a^mu"w"=4/3,a^mug=3/2)`
A ray of unpolarised light is incident on the surface of glass plate of µ = 1.54 at polarising angle, then angle of refraction is
Three immiscible liquids of densities d1 > d2 > d3 and refractive indices µ1 > µ2 > µ3 are put in a beaker. The height of each liquid column is `h/3`. A dot is made at the bottom of the beaker. For near normal vision, find the apparent depth of the dot.
A convex lens made of material of refractive index 1.5 and having a focal length of 10 cm is immersed in a liquid of refractive index 3.0. The lens will behave as ______.
A beam of light travels from air into a medium. Its speed and wavelength in the medium are 1.5 × 108 ms-1 and 230 nm respectively. The wavelength of light in the air will be ______.
