Advertisements
Advertisements
प्रश्न
Find the ratio in which the pint (-3, k) divide the join of A(-5, -4) and B(-2, 3),Also, find the value of k.
उत्तर
Let the point P (-3, k) divide the line AB in the ratio s : 1
Then, by the section formula :
`x = (mx_1 + nx_1) /(m+n) , y= (my_2 +ny_1)/(m+n)`
The coordinates of P are (-3,k).
`-3=(-2s-5)/(s+1) , k =(3s-4)/(s+1)`
⇒-3s-3=-2s-5,k(s+1)=3s-4
⇒-3s+2s=-5+3,k(s+1) = 3s-4
⇒-s=-2,k(s+1)= 3s-4
⇒ s =2,k(s+1)=3s-4
Therefore, the point P divides the line AB in the ratio 2 : 1.
Now, putting the value of s in the equation k(s+1)=3s-4 , we get:
k(2+1)=3(2)-4
⇒ 3k=6-4
⇒ 3k = 2⇒k=`2/3`
Therefore, the value of k`= 2/3`
That is, the coordinates of P are `(-3,2/3)`
APPEARS IN
संबंधित प्रश्न
On which axis do the following points lie?
P(5, 0)
Find the value of x such that PQ = QR where the coordinates of P, Q and R are (6, -1), (1, 3) and (x, 8) respectively.
Prove that (4, 3), (6, 4) (5, 6) and (3, 5) are the angular points of a square.
If the points A(4,3) and B( x,5) lie on the circle with center O(2,3 ) find the value of x .
If the point P(x, 3) is equidistant from the point A(7, −1) and B(6, 8), then find the value of x and find the distance AP.
If (0, −3) and (0, 3) are the two vertices of an equilateral triangle, find the coordinates of its third vertex.
Show that A (−3, 2), B (−5, −5), C (2,−3), and D (4, 4) are the vertices of a rhombus.
The points \[A \left( x_1 , y_1 \right) , B\left( x_2 , y_2 \right) , C\left( x_3 , y_3 \right)\] are the vertices of ΔABC .
(i) The median from A meets BC at D . Find the coordinates of the point D.
(ii) Find the coordinates of the point P on AD such that AP : PD = 2 : 1.
(iii) Find the points of coordinates Q and R on medians BE and CF respectively such thatBQ : QE = 2 : 1 and CR : RF = 2 : 1.
(iv) What are the coordinates of the centropid of the triangle ABC ?
If A(−3, 5), B(−2, −7), C(1, −8) and D(6, 3) are the vertices of a quadrilateral ABCD, find its area.
Find the value of k if points A(k, 3), B(6, −2) and C(−3, 4) are collinear.
If the mid-point of the segment joining A (x, y + 1) and B (x + 1, y + 2) is C \[\left( \frac{3}{2}, \frac{5}{2} \right)\] , find x, y.
If P (2, 6) is the mid-point of the line segment joining A(6, 5) and B(4, y), find y.
If P (x, 6) is the mid-point of the line segment joining A (6, 5) and B (4, y), find y.
Find the area of triangle with vertices ( a, b+c) , (b, c+a) and (c, a+b).
If the centroid of the triangle formed by the points (a, b), (b, c) and (c, a) is at the origin, then a3 + b3 + c3 =
If (−2, 1) is the centroid of the triangle having its vertices at (x , 0) (5, −2), (−8, y), then x, y satisfy the relation
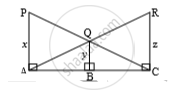
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
Points (1, –1) and (–1, 1) lie in the same quadrant.
Find the coordinates of the point which lies on x and y axes both.
Find the coordinates of the point whose abscissa is 5 and which lies on x-axis.
