Advertisements
Advertisements
प्रश्न
From a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2
[use `pi = 22/7`]
उत्तर
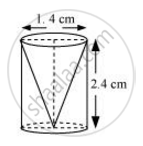
Given that,
Height (h) of the conical part = Height (h) of the cylindrical part = 2.4 cm
Diameter of the cylindrical part = 1.4 cm
Therefore, radius (r) of the cylindrical part = 0.7 cm
Slant height (l) of conical part =` sqrt(r^2 + h^2)`
`= sqrt((0.7)^2+(2.4)^2) `
`=sqrt(0.49+5.76)`
`=sqrt(6.25)`
= 2.5
Total surface area of the remaining solid will be = CSA of cylindrical part + CSA of conical part + Area of cylindrical base
= 2πrh + πrl + πr2
`= 2xx 22/7xx 0.7xx2.4+22/7xx0.7xx2.5+22/7xx0.7xx0.7`
= `4.4 xx 2.4 + 2.2 xx 2.5 + 2.2 xx 0.7`
= 10.56 + 5.50 +1.54
= 17.60 cm2
The total surface area of the remaining solid to the nearest cm2 is 18 cm2.
APPEARS IN
संबंधित प्रश्न
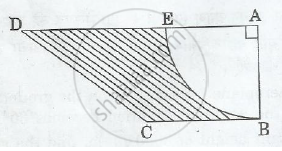
In Figure 2, ABCD is a trapezium of area 24.5 sq. cm. In it, AD|| BC, ∠ DAB = 900, AD = 10 cm and BC = 4 cm. If ABE is a quadrant of a circle, find the area of the shaded region. [Take π=22/7]
A solid wooden toy is in the form of a hemisphere surrounded by a cone of same radius. The radius of hemisphere is 3.5 cm and the total wood used in the making of toy is 166 `5/6` cm3. Find the height of the toy. Also, find the cost of painting the hemispherical part of the toy at the rate of Rs 10 per cm2 .[Use`pi=22/7`]
2 cubes each of volume 64 cm3 are joined end to end. Find the surface area of the resulting cuboid.
A hemispherical depression is cut out from one face of a cubical wooden block such that the diameter l of the hemisphere is equal to the edge of the cube. Determine the surface area of the remaining solid.
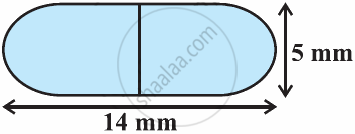
A medicine capsule is in the shape of cylinder with two hemispheres stuck to each of its ends (see the given figure). The length of the entire capsule is 14 mm and the diameter of the capsule is 5 mm. Find its surface area. [Use π = `22/7`]
Prove that the surface area of a sphere is equal to the curved surface area of the circumference cylinder__?
A solid is in the form of a right circular cylinder, with a hemisphere at one end and a cone at the other end. The radius of the common base is 3.5 cm and the heights of the cylindrical and conical portions are 10 cm. and 6 cm, respectively. Find the total surface area of the solid. (Use π =`22/7`)
A toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The radius and height of the cylindrical part are 5 cm and 13 cm respectively.The radii of the hemispherical and conical parts are the same as that of the cylindrical part.Find the surface area of the toy if the total height of the toy is 30 cm.
If the radii of circular ends of a bucket 24cm high are 5cm and 15cm. find surface area of
bucket?
If the radius of the base of a right circular cylinder is halved, keeping the height the same, then the ratio of the volume of the cylinder thus obtained to the volume of original cylinder is:
A solid cuboid of iron with dimensions 53 cm ⨯ 40 cm ⨯ 15 cm is melted and recast into a cylindrical pipe. The outer and inner diameters of pipe are 8 cm and 7 cm respectively. Find the length of pipe.
A solid metal sphere of 6 cm diameter is melted and a circular sheet of thickness 1 cm is prepared. Determine the diameter of the sheet.
Find the volume of a solid in the form of a right circular cylinder with hemi-spherical ends whose total length is 2.7 m and the diameter of each hemi-spherical end is 0.7 m.
Find the ratio of the volume of a cube to that of a sphere which will fit inside it.
The volume of a hemisphere is 19404 cm3. The total surface area of the hemisphere is
Match the following columns:
| Column I | Column II |
| (a) The radii of the circular ends of a bucket, in the form of the frustum of a cone of height 30 cm, are 20 cm and 10 cm respectively. The capacity of the bucket is ........cm3. |
(p) 2418π |
| (b) The radii of the circular ends of a conical bucket of height 15 cm are 20 and 12 cm respectively. The slant height of the bucket is ........ cm. |
(q) 22000 |
| (c) The radii of the circular ends of a solid frustum of a cone are 33 cm and 27 cm and its slant height is 10 cm. The total surface area of the bucket is .........cm2. |
(r) 12 |
| (d) Three solid metallic spheres of radii 3 cm, 4 cm and 5 cm are melted to form a single solid sphere. The diameter of the resulting sphere is ........ cm. |
(s) 17 |
A plumbline (sahul) is the combination of (see figure) ______.
3 cubes each of 8 cm edge are joined end to end. Find the total surface area of the cuboid.
A tent is in the shape of a cylinder surmounted by a conical top. If the height and radius of the cylindrical part are 3 m and 14 m respectively, and the total height of the tent is 13.5 m, find the area of the canvas required for making the tent, keeping a provision of 26 m2 of canvas for stitching and wastage. Also, find the cost of the canvas to be purchased at the rate of ₹ 500 per m2.
The ratio of total surface area of a solid hemisphere to the square of its radius is ______.
