Advertisements
Advertisements
प्रश्न
From an external point, two tangents are drawn to a circle. Prove that the line joining the external point to the centre of the circle bisects the angle between the two tangents.
उत्तर
Draw a circle with centre O and take an external point P.
PA and PB are the tangents.
Join OP.
Now in ΔOAP and ΔOBP,
OA = OB ...(Radius of circle)
OP = OP ...(Common)
PA = PB ...(Tangents are equal)
So, by S.S.S criteria,
ΔOAP ≅ ΔOBP
So, ∠APO = ∠BPO
Hence, OP bisects ∠APB.
APPEARS IN
संबंधित प्रश्न
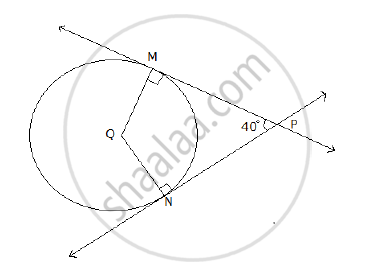
In the following figure, Q is the center of the circle. PM and PN are tangents to the circle. If ∠MPN = 40° , find ∠MQN.
In the given figure, XY and X’Y’ are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting XY at A and X’Y’ at B. Prove that ∠AOB = 90°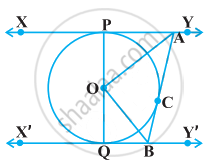
Prove that the centre of a circle touching two intersecting lines lies on the angle bisector of the lines.
In the figure given below, O is the center of the circle and SP is a tangent. If ∠SRT = 65°, find the value of x, y and Z.

A tangent is drawn from a point at a distance of 17 cm of circle C(0, r) of radius 8 cm. The length of its tangent is ______
The length of a tangent drawn from a point at a distance of 10 cm of the circle is 8 cm. The radius of the circle is ______
If from an external point B of a circle with centre O, two tangents BC and BD are drawn such that ∠DBC = 120°, prove that BC + BD = BO, i.e., BO = 2BC.
In the given figure, PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q meet at a point T. Find the length of TP.

In the given figure, perimeter of ΔPQR is 20 cm. Find the length of tangent PA.

Two concentric circles with centre O are of radii 3 cm and 5 cm. Find the length of chord AB of the larger circle which touches the smaller circle at P.

