Advertisements
Advertisements
प्रश्न
In the given figure, XY and X’Y’ are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting XY at A and X’Y’ at B. Prove that ∠AOB = 90°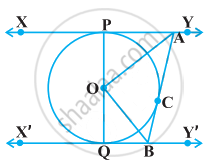
उत्तर १
Let us join point O to C.
In ΔOPA and ΔOCA,
OP = OC ...(Radii of the same circle)
AP = AC ......(Tangents from point A)
AO = AO ....(Common side)
ΔOPA ≅ ΔOCA (SSS congruence criterion)
Therefore, P ↔ C, A ↔ A, and O ↔ O
∠POA = ∠COA … (i)
Similarly, ΔOQB ≅ ΔOCB
∠QOB = ∠COB … (ii)
Since POQ is the diameter of the circle, it is a straight line.
Therefore, ∠POA + ∠COA + ∠COB + ∠QOB = 180º
From equations (i) and (ii), it can be observed that
2∠COA + 2 ∠COB = 180º
∠COA + ∠COB = 90º
∠AOB = 90°
उत्तर २
Given: XY and X'Y' are two parallel tangents to the circle with centre O touching the circle at P and Q, respectively. AB is a tangent at the point C, which intersects XY at A and X'Y' at B.
To prove: ∠AOB = 90°
Construction: Join OC.
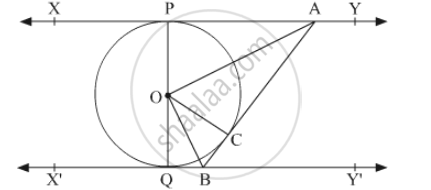
In ΔOAP and ΔOAC,
OP = OC ... (Radii of the same circle)
AP = AC. ...(Length of tangents drawn from an external point to a circle are equal)
AO = OA ....(Common side)
ΔOAP ≅ ΔOAC .....(SSS congruence criterion)
∴ ∠AOP = ∠COA ....(C.P.C.T) .....(1)
Similarly, ΔOBQ ≅ ΔOBC
∴ ∠BOQ = ∠COB .....(2)
POQ is a diameter of the circle. Hence, it is a straight line.
∴ ∠AOP + ∠COA + ∠BOQ + ∠COB = 180º
2∠COA + 2∠COB = 180º ... [From (1) and (2)]
⇒ ∠COA + ∠COB = 90º
⇒ ∠AOB = 90°
Hence proved.
APPEARS IN
संबंधित प्रश्न
Prove that “The lengths of the two tangent segments to a circle drawn from an external point are equal.”
In the following figure, Q is the centre of a circle and PM, PN are tangent segments to the circle. If ∠MPN = 50°, find ∠MQN.

If from an external point P of a circle with centre O, two tangents PQ and PR are drawn such that ∠QPR = 120°, prove that 2PQ = PO.
Prove that a parallelogram circumscribing a circle is a rhombus.
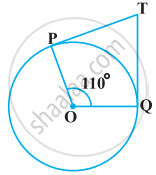
In the given figure, if TP and TQ are the two tangents to a circle with centre O so that ∠POQ = 110°, then ∠PTQ is equal to ______.
Prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
A triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 8 cm and 6 cm respectively (see given figure). Find the sides AB and AC.

In the following figure, PQ = QR, ∠RQP = 68°, PC and CQ are tangents to the circle with centre O.

Calculate the values of:
- ∠QOP
- ∠QCP
In fig. 6, l and m are two parallel tangents to a circle with centre O, touching the circle at A and B respectively. Another tangent at C intersects the line l at D and m at E. Prove that ∠DOE = 90° ?
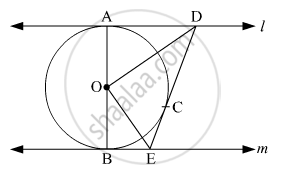
M and N are the midpoints of chords AB and CD . The line MN passes through the centre O . Prove that AB || CD.
In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.

Find: ∠AOB
The number of tangents drawn at a point of the circle is/are ______
The length of the tangent from an external point on a circle is ______
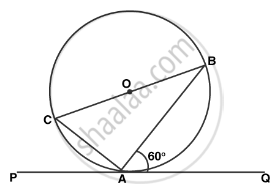
In the given figure, PAQ is the tangent. BC is the diameter of the circle. ∠BAQ = 60°, find ∠ABC.
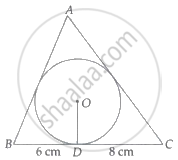
In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 6 cm and 8 cm respectively. If the area of ΔABC is 84 cm2, find the lengths of sides AB and AC.
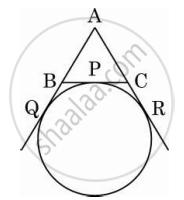
In the given figure, perimeter of ΔPQR is 20 cm. Find the length of tangent PA.

If a circle is touching the side BC of ΔABC at P and is touching AB and AC produced at Q and R respectively (see the figure). Prove that AQ = `1/2` (perimeter of ΔABC).
Two concentric circles with centre O are of radii 3 cm and 5 cm. Find the length of chord AB of the larger circle which touches the smaller circle at P.

