Advertisements
Advertisements
प्रश्न
How many triangles can be drawn having its angles as 45°, 64° and 72°? Give reason for your answer.
उत्तर
It is given that the triangle has its angles as 45°, 64°, and 72°.
On adding these, we get
Sum of three angles = 45° + 64° + 72°
⇒ Sum of three angles = 181°
The sum of three angles of the triangle should be equal to 180°.
Since, the sum of the given angles exceeds 180°, no such triangle exists with its angles as 45°, 64° and 72°.
APPEARS IN
संबंधित प्रश्न
Find the value of the unknown x in the following diagram:

Find the value of the unknown x in the following diagram:
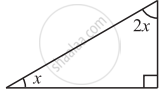
In the following triangle, find the value of x
Can a triangle have all angles less than 60°? Give reason for your answer.
In the following figure, DE || QR and AP and BP are bisectors of ∠EAB and ∠RBA, respectively. Find ∠APB.

If one of the angles of a triangle is 110°, then the angle between the bisectors of the other two angles is ______.
If one angle of a triangle is equal to the sum of other two, then the measure of that angle is ______.
It is possible to have a triangle in which two of the angles are right angles.
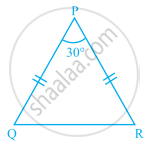
In ΔPQR of the given figure, PQ = PR. Find the measures of ∠Q and ∠R.
In a right-angled triangle if an angle measures 35°, then find the measure of the third angle.
