Advertisements
Advertisements
प्रश्न
Can a triangle have two obtuse angles? Give reason for your answer.
उत्तर
We know, the sum of three angles of a triangle is equal to 180°.
Also, an obtuse angle is one whose value is greater than 90° but less than 180°.
If the triangle has two obtuse angles, then there are at least two angles which are 91° each.
On adding these two angles,
Sum of the two angles = 91° + 91°
⇒ Sum of the two angles = 182°
This already exceeds the sum of three angles of the triangle, even without considering the third angle.
Again, if we consider the case where one angle of the triangle is 90°, we have a right-angled triangle. The rest two angles must be less than 90° each so as to satisfy the property of a triangle, which states that the sum of the three angles of a triangle should be equal to 180°.
Thus, a triangle cannot have two obtuse angles.
APPEARS IN
संबंधित प्रश्न
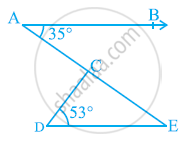
In the given figure, if AB || DE, ∠BAC = 35º and ∠CDE = 53º, find ∠DCE.
Find the value of the unknowns x and y in the following diagram:

Two line segments `bar("AD")` and `bar("BC")` intersect at O. Joining `bar("AB")` and `bar("DC")` we get two triangles, ∆AOB and ∆DOC as shown in the figure. Find the ∠A and ∠B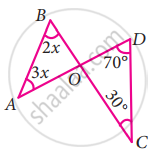
The angles of a triangle are in the ratio 5 : 3 : 7. The triangle is ______.
If one of the angles of a triangle is 130°, then the angle between the bisectors of the other two angles can be ______.
Bisectors of interior ∠B and exterior ∠ACD of a ∆ABC intersect at the point T. Prove that `∠BTC = 1/2 ∠BAC`.
In a right-angled triangle, the angles other than the right angle are ______.
In an isosceles triangle, one angle is 70°. The other two angles are of ______.
- 55° and 55°
- 70° and 40°
- any measure
In the given option(s) which of the above statement(s) are true?
If one of the angles of a triangle is 110°, then the angle between the bisectors of the other two angles is ______.
In a triangle ABC, the measure of angle A is 40° less than the measure of angle B and 50° less than that of angle C. Find the measure of ∠A.
