Advertisements
Advertisements
Question
Can a triangle have two obtuse angles? Give reason for your answer.
Solution
We know, the sum of three angles of a triangle is equal to 180°.
Also, an obtuse angle is one whose value is greater than 90° but less than 180°.
If the triangle has two obtuse angles, then there are at least two angles which are 91° each.
On adding these two angles,
Sum of the two angles = 91° + 91°
⇒ Sum of the two angles = 182°
This already exceeds the sum of three angles of the triangle, even without considering the third angle.
Again, if we consider the case where one angle of the triangle is 90°, we have a right-angled triangle. The rest two angles must be less than 90° each so as to satisfy the property of a triangle, which states that the sum of the three angles of a triangle should be equal to 180°.
Thus, a triangle cannot have two obtuse angles.
APPEARS IN
RELATED QUESTIONS
In the following triangle, find the value of x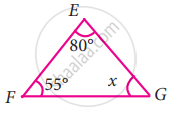
In ∆ABC, if ∠B is 3 times ∠A and ∠C is 2 times ∠A, then find the angle
How many triangles can be drawn having its angles as 53°, 64° and 63°? Give reason for your answer.
Two adjacent angles are equal. Is it necessary that each of these angles will be a right angle? Justify your answer.
In the given figure, ∠BAC = 90°, AD ⊥ BC and ∠BAD = 50°, then ∠ACD is ______.

If one angle of a triangle is equal to the sum of the other two angles, the triangle is ______.
The measure of three angles of a triangle are in the ratio 5:3:1. Find the measures of these angles.
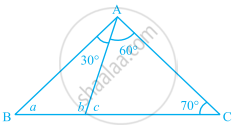
In the given figure, find the values of a, b and c.
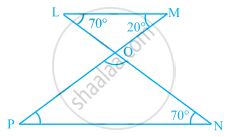
In the given figure, find the measures of ∠PON and ∠NPO.
In a right-angled triangle if an angle measures 35°, then find the measure of the third angle.
