Advertisements
Advertisements
प्रश्न
If ΔABC ~ ΔEDF and ΔABC is not similar to ΔDEF, then which of the following is not true?
विकल्प
BC . EF = AC . FD
AB . EF = AC . DE
BC . DE = AB . EF
BC . DE = AB . FD
उत्तर
BC . DE = AB . EF
Explanation:
We know that,
If sides of one triangle are proportional to the side of the other triangle and the corresponding angles are also equal, then the triangles are similar by SSS similarity.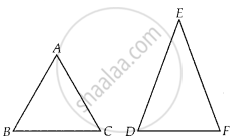
So, ∆ABC ∼ ∆EDF
Using similarity property,
`("AB")/("ED") = ("BC")/("DF") = ("AC")/("EF")`
Taking `("AB")/("ED") = ("BC")/("DF")`, we get
`("AB")/("ED") = ("BC")/("DF")`
AB . DF = ED . BC
So, option (d) BC . DE = AB . FD is true
Taking `("BC")/("DF") = ("AC")/("EF")`, we get
`("BC")/("DF") = ("AC")/("EF")`
⇒ BC . EF = AC . DF
So, option (a) BC . EF = AC . FD is true
Taking `("AB")/("ED") = ("AC")/("EF")`, we get,
`("AB")/("ED") = ("AC")/("EF")`
AB . EF = ED . AC
So, option (b) AB . EF = AC . DE is true.
APPEARS IN
संबंधित प्रश्न
State which pair of triangles in the given figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
In the following figure, altitudes AD and CE of ΔABC intersect each other at the point P. Show that:

ΔABD ∼ ΔCBE
CD and GH are, respectively, the bisectors of ∠ACB and ∠EGF such that D and H lie on sides AB and FE of ΔABC and ΔEFG, respectively. If ΔABC ~ ΔFEG, Show that
- `("CD")/("GH") = ("AC")/("FG")`
- ΔDCB ~ ΔHGE
- ΔDCA ~ ΔHGF
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR, prove that `("AB")/("PQ") = ("AD")/("PM")`.
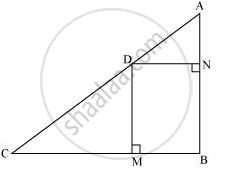
In the given figure, D is a point on hypotenuse AC of ΔABC, DM ⊥ BC and DN ⊥ AB, Prove that:
(i) DM2 = DN.MC
(ii) DN2 = DM.AN
In the following Figure, ∠ABC = 90° and BD ⊥ AC. If BD = 8 cm and AD = 4 cm, find CD.

In figure, if ∠1 = ∠2 and ΔNSQ ≅ ΔMTR, then prove that ΔPTS ~ ΔPRQ.
If in two triangles ABC and PQR, `(AB)/(QR) = (BC)/(PR) = (CA)/(PQ)`, then ______.
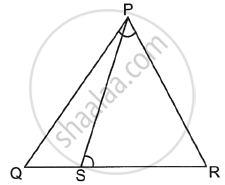
In the given figure, S is a point on side QR of ΔPQR such that ∠QPR = ∠PSR. Use this information to prove that PR2 = QR × SR.
If in two right triangles, one of the acute angles of one triangle is equal to an acute angle of the other triangle, can you say that the two triangles will be similar? Why?
