Advertisements
Advertisements
Question
If ΔABC ~ ΔEDF and ΔABC is not similar to ΔDEF, then which of the following is not true?
Options
BC . EF = AC . FD
AB . EF = AC . DE
BC . DE = AB . EF
BC . DE = AB . FD
Solution
BC . DE = AB . EF
Explanation:
We know that,
If sides of one triangle are proportional to the side of the other triangle and the corresponding angles are also equal, then the triangles are similar by SSS similarity.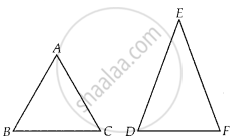
So, ∆ABC ∼ ∆EDF
Using similarity property,
`("AB")/("ED") = ("BC")/("DF") = ("AC")/("EF")`
Taking `("AB")/("ED") = ("BC")/("DF")`, we get
`("AB")/("ED") = ("BC")/("DF")`
AB . DF = ED . BC
So, option (d) BC . DE = AB . FD is true
Taking `("BC")/("DF") = ("AC")/("EF")`, we get
`("BC")/("DF") = ("AC")/("EF")`
⇒ BC . EF = AC . DF
So, option (a) BC . EF = AC . FD is true
Taking `("AB")/("ED") = ("AC")/("EF")`, we get,
`("AB")/("ED") = ("AC")/("EF")`
AB . EF = ED . AC
So, option (b) AB . EF = AC . DE is true.
APPEARS IN
RELATED QUESTIONS
S and T are point on sides PR and QR of ΔPQR such that ∠P = ∠RTS. Show that ΔRPQ ∼ ΔRTS.
In the following figure, altitudes AD and CE of ΔABC intersect each other at the point P. Show that:
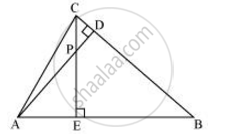
ΔAEP ∼ ΔCDP
In the following figure, ABC and AMP are two right triangles, right-angled at B and M respectively, prove that:

- ΔABC ~ ΔAMP
- `("CA")/("PA") = ("BC")/("MP")`
In ΔABC, AL and CM are the perpendiculars from the vertices A and C to BC and AB respectively. If AL and CM intersect at O, prove that:
(i) ΔOMA and ΔOLC
(ii) `"OA"/"OC"="OM"/"OL"`
State the AAA-similarity criterion
A ladder 10m long reaches the window of a house 8m above the ground. Find the distance of the foot of the ladder from the base of the wall.
In the given figure, ∠AMN = ∠MBC = 76° . If p, q and r are the lengths of AM, MB and BC respectively then express the length of MN of terms of P, q and r.

In the given figure, value of x(in cm) is

It is given that ΔABC ~ ΔDFE, ∠A =30°, ∠C = 50°, AB = 5 cm, AC = 8 cm and DF = 7.5 cm. Then, the following is true ______.

In the above figure, seg AC and seg BD intersect each other in point P. If `("AP")/("CP") = ("BP")/("DP")`, then complete the following activity to prove ΔABP ∼ ΔCDP.
Activity: In ΔABP and ΔCDP
`("AP")/("CP") = ("BP")/("DP")` ......`square`
∴ ∠APB ≅ `square` ......Vertically opposite angles
∴ `square` ∼ ΔCDP ....... `square` test of similarity.
