Question

If ${\displaystyle \cos \theta =\frac{12}{13}}$, show that ${\displaystyle \sin \theta (1-\tan \theta )=\frac{35}{156}}$

Answer 1

Given: cos θ = ${\displaystyle \frac{12}{13}}$

To prove: sin θ (1 − tan θ) = ${\displaystyle \frac{35}{156}}$

Proof: we know, cos θ = ${\displaystyle \frac{B}{H}}$

where the right-angled triangle's base is B and its hypotenuse is H. ∠ACB = 8 is achieved by building a right triangle ABC at a right angle to B. 

AB is perpendicular, BC = 12 is base, and AC = 13 is hypotenuse. According to Pythagoras theorem, we have

AC² = AB² + BC²

13² = AB² + 12²

169 = AB² + 144

169 − 144 = AB²

25 = AB²

AB = ${\displaystyle \sqrt{25}}$ = 5

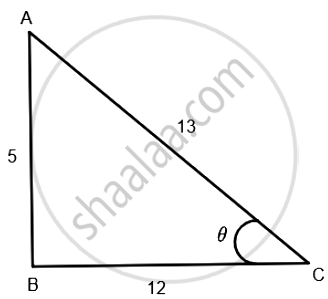
sin θ = ${\displaystyle \frac{P}{H}=\frac{5}{13}}$

So, tan θ = ${\displaystyle \frac{P}{H}=\frac{5}{12}}$

Put the values in sin θ(1 − tanθ) to find its value,

sinθ(1 − tanθ) = ${\displaystyle \frac{15}{3}(1-\frac{5}{12})}$

= ${\displaystyle \frac{5}{13}\times \frac{7}{12}=\frac{35}{156}}$

Hence Proved.