Advertisements
Advertisements
प्रश्न
In ΔABC right angled at B, AB = 24 cm, BC = 7 m. Determine:
sin A, cos A
उत्तर
ΔABC is right angled at B
AB = 24 cm, BC = 7 cm
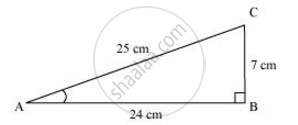
Let ‘x’ be the hypotenuse,
By applying Pythagoras
AC2 = AB2 + BC2
x2 = 242 + 72
x2 = 576 + 49
x2 = 625
x = 25
For Sin A, Cos A
At ∠A, opposite side = 7
adjacent side = 24
hypotenuse = 25
sin A = `"opposite side"/"hypotenuse" =("BC")/("AC") = 7/25`
cos A = `"adjacent side"/"hypotenuse" = ("AB")/("AC") = 24/25`
संबंधित प्रश्न
Given sec θ = `13/12`, calculate all other trigonometric ratios.
State whether the following are true or false. Justify your answer.
The value of tan A is always less than 1.
State whether the following are true or false. Justify your answer.
sin θ = `4/3`, for some angle θ.
If 4 tan θ = 3, evaluate `((4sin theta - cos theta + 1)/(4sin theta + cos theta - 1))`
If 3 tan θ = 4, find the value of `(4cos theta - sin theta)/(2cos theta + sin theta)`
If tan θ = `a/b` prove that `(a sin theta - b cos theta)/(a sin theta + b cos theta) = (a^2 - b^2)/(a^2 + b^2)`
If `tan theta = 1/sqrt7` `(cosec^2 theta - sec^2 theta)/(cosec^2 theta + sec^2 theta) = 3/4`
Evaluate the following
cos2 30° + cos2 45° + cos2 60° + cos2 90°
Evaluate the following
`sin^2 30° cos^2 45 ° + 4 tan^2 30° + 1/2 sin^2 90° − 2 cos^2 90° + 1/24 cos^2 0°`
Evaluate the Following
(cos 0° + sin 45° + sin 30°)(sin 90° − cos 45° + cos 60°)
Find the value of x in each of the following :
cos x = cos 60º cos 30º + sin 60º sin 30º
The value of sin² 30° – cos² 30° is ______.
If cos (40° + A) = sin 30°, then value of A is ______.
`(sin theta)/(1 + cos theta)` is ______.
If sin θ + sin² θ = 1, then cos² θ + cos4 θ = ______.
A ladder rests against a vertical wall at an inclination α to the horizontal. Its foot is pulled away from the wall through a distance p so that its upper end slides a distance q down the wall and then the ladder makes an angle β to the horizontal. Show that `p/q = (cos β - cos α)/(sin α - sin β)`
Prove that: cot θ + tan θ = cosec θ·sec θ
Proof: L.H.S. = cot θ + tan θ
= `square/square + square/square` ......`[∵ cot θ = square/square, tan θ = square/square]`
= `(square + square)/(square xx square)` .....`[∵ square + square = 1]`
= `1/(square xx square)`
= `1/square xx 1/square`
= cosec θ·sec θ ......`[∵ "cosec" θ = 1/square, sec θ = 1/square]`
= R.H.S.
∴ L.H.S. = R.H.S.
∴ cot θ + tan θ = cosec·sec θ
Find will be the value of cos 90° + sin 90°.
If sin θ + cos θ = `sqrt(2)` then tan θ + cot θ = ______.
