Advertisements
Advertisements
Question
In ΔABC right angled at B, AB = 24 cm, BC = 7 m. Determine:
sin A, cos A
Solution
ΔABC is right angled at B
AB = 24 cm, BC = 7 cm
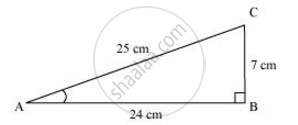
Let ‘x’ be the hypotenuse,
By applying Pythagoras
AC2 = AB2 + BC2
x2 = 242 + 72
x2 = 576 + 49
x2 = 625
x = 25
For Sin A, Cos A
At ∠A, opposite side = 7
adjacent side = 24
hypotenuse = 25
sin A = `"opposite side"/"hypotenuse" =("BC")/("AC") = 7/25`
cos A = `"adjacent side"/"hypotenuse" = ("AB")/("AC") = 24/25`
APPEARS IN
RELATED QUESTIONS
If ∠A and ∠B are acute angles such that cos A = cos B, then show that ∠A = ∠B.
In ΔPQR, right angled at Q, PR + QR = 25 cm and PQ = 5 cm. Determine the values of sin P, cos P and tan P.
State whether the following are true or false. Justify your answer.
The value of tan A is always less than 1.
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sin theta = 11/5`
If `tan theta = a/b`, find the value of `(cos theta + sin theta)/(cos theta - sin theta)`
If sec θ = `13/5, "show that" (2sinθ - 3 cosθ)/(4sinθ - 9cosθ) = 3`.
If `cot theta = 1/sqrt3` show that `(1 - cos^2 theta)/(2 - sin^2 theta) = 3/5`
if `sin theta = 3/4` prove that `sqrt(cosec^2 theta - cot)/(sec^2 theta - 1) = sqrt7/3`
If Cosec A = 2 find `1/(tan A) + (sin A)/(1 + cos A)`
Evaluate the following
sin2 30° + sin2 45° + sin2 60° + sin2 90°
Evaluate the following
cos2 30° + cos2 45° + cos2 60° + cos2 90°
Evaluate the following
tan2 30° + tan2 60° + tan2 45°
Evaluate the following
`sin^2 30° cos^2 45 ° + 4 tan^2 30° + 1/2 sin^2 90° − 2 cos^2 90° + 1/24 cos^2 0°`
Evaluate the Following
`(sin 30^@ - sin 90^2 + 2 cos 0^@)/(tan 30^@ tan 60^@)`
Find the value of x in the following :
`2sin 3x = sqrt3`
If A and (2A – 45°) are acute angles such that sin A = cos (2A – 45°), then tan A is equal to ______.
In the given figure, if sin θ = `7/13`, which angle will be θ?

If θ is an acute angle of a right angled triangle, then which of the following equation is not true?
Evaluate: 5 cosec2 45° – 3 sin2 90° + 5 cos 0°.
