Advertisements
Advertisements
Question
If `sec θ = 13/5`, show that `(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ) = 3`.
Advertisements
Solution 1
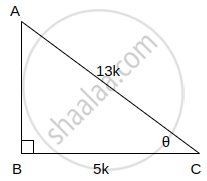
Given: `sec θ = 13/5`
We know that,
sec θ = `"Hypotenuse"/"Adjacent Side"`
sec θ = `13/5 = "AC"/"BC"`
Let AC = 13k and BC = 5k
In ΔABC, ∠B = 90°
By Pythagoras theorem,
AC2 = AB2 + BC2
(13k)2 = AB2 + (5k)2
AB2 = 169k2 – 25k2
AB2 = 144k2
AB = 12k
sin θ = `"AB"/"AC" = "12k"/"13k" = 12/13`
cos θ = `"BC"/"AC" = "5k"/"13k" = 5/13`
LHS = `(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ)`
LHS = `[2 × (12/13) - 3 × (5/13)]/[4 × (12/13) - 9 × (5/13)]`
LHS = `[24/13 - 15/13]/[48/13 + 45/13]`
LHS = `[9/13]/[3/13]`
LHS = `9/(cancel13) × cancel13/3`
LHS = `9/3`
LHS = 3
RHS = 3
LHS = RHS
`(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ) = 3`
Hence proved.
Solution 2
Given: sec θ = `13/5`
cos θ = `1/secθ = 5/13`
sin2θ = 1 – cos2θ
sin2θ = `1 - (5/13)^2`
sin2θ = `1 - 25/169`
sin2θ = `(169 − 25)/169`
sin2θ = `144/169`
sin θ = `12/13`
Now, put the values in the equation,
LHS = `(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ)`
LHS = `(2 × (12/13) - 3 × (5/13))/(4 × (12/13) - 9 × (5/13))`
LHS = `(24/13 - 15/13)/(48/13 - 45/13)`
LHS = `((24- 15)/cancel13)/((48 - 45)/cancel13)`
LHS = `9/3`
LHS = 3
RHS = 3
LHS = RHS
`(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ) = 3`
Hence proved.
RELATED QUESTIONS
In ΔABC right angled at B, AB = 24 cm, BC = 7 m. Determine:
sin C, cos C
State whether the following are true or false. Justify your answer.
The value of tan A is always less than 1.
State whether the following are true or false. Justify your answer.
cos A is the abbreviation used for the cosecant of angle A.
If 4 tan θ = 3, evaluate `((4sin theta - cos theta + 1)/(4sin theta + cos theta - 1))`
Prove that `(sin "A" - 2sin^3 "A")/(2cos^3 "A" - cos "A") = tan "A"`
In the following, one of the six trigonometric ratios is given. Find the values of the other trigonometric ratios.
`tan theta = 8/15`
If 3 cot θ = 2, find the value of `(4sin theta - 3 cos theta)/(2 sin theta + 6cos theta)`.
If tan θ = `a/b` prove that `(a sin theta - b cos theta)/(a sin theta + b cos theta) = (a^2 - b^2)/(a^2 + b^2)`
if `cos theta = 3/5`, find the value of `(sin theta - 1/(tan theta))/(2 tan theta)`
If `tan theta = 24/7`, find that sin θ + cos θ.
if `sin theta = 3/4` prove that `sqrt(cosec^2 theta - cot)/(sec^2 theta - 1) = sqrt7/3`
Evaluate the following
`2 sin^2 30^2 - 3 cos^2 45^2 + tan^2 60^@`
Evaluate the Following
`(sin 30^@ - sin 90^2 + 2 cos 0^@)/(tan 30^@ tan 60^@)`
Evaluate the Following:
`tan 45^@/(cosec 30^@) + sec 60^@/cot 45^@ - (5 sin 90^@)/(2 cos 0^@)`
Find the value of x in the following :
`sqrt3 sin x = cos x`
Find the value of x in the following :
`sqrt3 tan 2x = cos 60^@ + sin45^@ cos 45^@`
Find the value of x in the following :
cos 2x = cos 60° cos 30° + sin 60° sin 30°
sin (45° + θ) – cos (45° – θ) is equal to ______.
If cos (40° + A) = sin 30°, then value of A is ______.
5 tan² A – 5 sec² A + 1 is equal to ______.
If cos A = `4/5`, then the value of tan A is ______.
Find the value of sin 45° + cos 45° + tan 45°.
In the given figure, if sin θ = `7/13`, which angle will be θ?
`sqrt(3)` cos2A + `sqrt(3)` sin2A is equal to ______.
If sin θ + cos θ = `sqrt(2)` then tan θ + cot θ = ______.
If sinθ = `1/sqrt(2)` and `π/2 < θ < π`. Then the value of `(sinθ + cosθ)/tanθ` is ______.
(3 sin2 30° – 4 cos2 60°) is equal to ______.
In ΔBC, right angled at C, if tan A = `8/7`, then the value of cot B is ______.
Evaluate: 5 cosec2 45° – 3 sin2 90° + 5 cos 0°.
