Advertisements
Advertisements
प्रश्न
If `sec θ = 13/5`, show that `(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ) = 3`.
Advertisements
उत्तर १
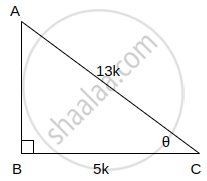
Given: `sec θ = 13/5`
We know that,
sec θ = `"Hypotenuse"/"Adjacent Side"`
sec θ = `13/5 = "AC"/"BC"`
Let AC = 13k and BC = 5k
In ΔABC, ∠B = 90°
By Pythagoras theorem,
AC2 = AB2 + BC2
(13k)2 = AB2 + (5k)2
AB2 = 169k2 – 25k2
AB2 = 144k2
AB = 12k
sin θ = `"AB"/"AC" = "12k"/"13k" = 12/13`
cos θ = `"BC"/"AC" = "5k"/"13k" = 5/13`
LHS = `(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ)`
LHS = `[2 × (12/13) - 3 × (5/13)]/[4 × (12/13) - 9 × (5/13)]`
LHS = `[24/13 - 15/13]/[48/13 + 45/13]`
LHS = `[9/13]/[3/13]`
LHS = `9/(cancel13) × cancel13/3`
LHS = `9/3`
LHS = 3
RHS = 3
LHS = RHS
`(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ) = 3`
Hence proved.
उत्तर २
Given: sec θ = `13/5`
cos θ = `1/secθ = 5/13`
sin2θ = 1 – cos2θ
sin2θ = `1 - (5/13)^2`
sin2θ = `1 - 25/169`
sin2θ = `(169 − 25)/169`
sin2θ = `144/169`
sin θ = `12/13`
Now, put the values in the equation,
LHS = `(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ)`
LHS = `(2 × (12/13) - 3 × (5/13))/(4 × (12/13) - 9 × (5/13))`
LHS = `(24/13 - 15/13)/(48/13 - 45/13)`
LHS = `((24- 15)/cancel13)/((48 - 45)/cancel13)`
LHS = `9/3`
LHS = 3
RHS = 3
LHS = RHS
`(2 sin θ - 3 cos θ)/(4 sin θ - 9 cos θ) = 3`
Hence proved.
APPEARS IN
संबंधित प्रश्न
If sin A = `3/4`, calculate cos A and tan A.
If ∠A and ∠B are acute angles such that cos A = cos B, then show that ∠A = ∠B.
State whether the following are true or false. Justify your answer.
sin θ = `4/3`, for some angle θ.
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sec theta = 13/5`
In the following, one of the six trigonometric ratios is given. Find the values of the other trigonometric ratios.
`cos theta = 12/2`
If `tan theta = a/b`, find the value of `(cos theta + sin theta)/(cos theta - sin theta)`
If `cos θ = 12/13`, show that `sin θ (1 - tan θ) = 35/156`.
if `cot theta = 3/4` prove that `sqrt((sec theta - cosec theta)/(sec theta +cosec theta)) = 1/sqrt7`
If `tan theta = 24/7`, find that sin 𝜃 + cos 𝜃
if `sin theta = 3/4` prove that `sqrt(cosec^2 theta - cot)/(sec^2 theta - 1) = sqrt7/3`
Evaluate the following
sin 45° sin 30° + cos 45° cos 30°
Evaluate the following
cos 60° cos 45° - sin 60° ∙ sin 45°
Evaluate the following
cos2 30° + cos2 45° + cos2 60° + cos2 90°
Evaluate the following
tan2 30° + tan2 60° + tan2 45°
Evaluate the Following
`4/(cot^2 30^@) + 1/(sin^2 60^@) - cos^2 45^@`
Evaluate the Following
4(sin4 30° + cos2 60°) − 3(cos2 45° − sin2 90°) − sin2 60°
Evaluate the Following
`sin 30^2/sin 45^@ + tan 45^@/sec 60^@ - sin 60^@/cot 45^@ - cos 30^@/sin 90^@`
Find the value of x in the following :
cos 2x = cos 60° cos 30° + sin 60° sin 30°
In ΔABC is a right triangle such that ∠C = 90° ∠A = 45°, BC = 7 units find ∠B, AB and AC
If cosec θ - cot θ = `1/3`, the value of (cosec θ + cot θ) is ______.
If cos A + cos² A = 1, then sin² A + sin4 A is equal to ______.
`(sin theta)/(1 + cos theta)` is ______.
Prove the following:
If tan A = `3/4`, then sinA cosA = `12/25`
In the given figure, if sin θ = `7/13`, which angle will be θ?
`sqrt(3)` cos2A + `sqrt(3)` sin2A is equal to ______.
If cos(α + β) = `(3/5)`, sin(α – β) = `5/13` and 0 < α, β < `π/4`, then tan (2α) is equal to ______.
The maximum value of the expression 5cosα + 12sinα – 8 is equal to ______.
If sinθ = `1/sqrt(2)` and `π/2 < θ < π`. Then the value of `(sinθ + cosθ)/tanθ` is ______.
(3 sin2 30° – 4 cos2 60°) is equal to ______.
