Advertisements
Advertisements
प्रश्न
Given that sinα = `1/2` and cosβ = `1/2`, then the value of (α + β) is ______.
विकल्प
0°
30°
60°
90°
उत्तर
Given that sinα = `1/2` and cosβ = `1/2`, then the value of (α + β) is 90°.
Explanation:
Given,
sinα = `1/2` = sin30° ...`[∵ sin 30^circ = 1/2]`
⇒ α = 30°
And cosβ = `1/2` = cos60° ...`[∵ 60^circ = 1/2]`
⇒ β = 60°
∴ α + β = 30° + 60° = 90°
APPEARS IN
संबंधित प्रश्न
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sin theta = 11/5`
if `sec A = 17/8` verify that `(3 - 4sin^2A)/(4 cos^2 A - 3) = (3 - tan^2 A)/(1 - 3 tan^2 A)`
if `cot theta = 3/4` prove that `sqrt((sec theta - cosec theta)/(sec theta +cosec theta)) = 1/sqrt7`
If `sin theta = a/b` find sec θ + tan θ in terms of a and b.
If `tan θ = 20/21` show that `(1 - sin theta + cos theta)/(1 + sin theta + cos theta) = 3/7`
sin (45° + θ) – cos (45° – θ) is equal to ______.
Prove the following:
If tan A = `3/4`, then sinA cosA = `12/25`
In ΔABC, ∠ABC = 90° and ∠ACB = θ. Then write the ratios of sin θ and tan θ from the figure.
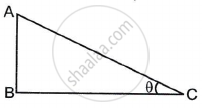
Find will be the value of cos 90° + sin 90°.
Find an acute angle θ when `(cos θ - sin θ)/(cos θ + sin θ) = (1 - sqrt(3))/(1 + sqrt(3))`
