Advertisements
Advertisements
प्रश्न
If sin θ = `12/13`, Find `(sin^2 θ - cos^2 θ)/(2sin θ cos θ) × 1/(tan^2 θ)`.
Advertisements
उत्तर
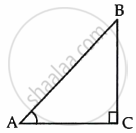
Given: Sin θ = `12/13 = "AB"/"AC"`
Let, AB = 12k and AC = 13k
In ΔABC, ∠B = 90°
By pythagoras theorem,
AB2 + BC2 = AC2
(12k)2 + BC2 = (13k)2
144k2 + BC2 = 169k2
BC2 = 169k2 - 144k2
BC2 = 25k2
Taking square root,
BC = 5k
∴ Cos θ = `"BC"/"AC" = "5k"/"13k" = 5/13`
∴ tan θ = `"AB"/"BC" = "12k"/"5k" = 12/5`
Now,
`(sin^2 θ - cos^2 θ)/(2sin θ cos θ) × 1/(tan^2 θ)`.
⇒ `[(12/13)^2 - (5/13)^2]/[2 × 12/13 × 5/13] × 1/(12/5)^2`
⇒ `[(144/169) - (25/169)]/[120/169] × 1/(144/25)`
⇒ `[(144/169) - (25/169)]/[120/169] × 25/144`
⇒ `((144 - 25)/cancel169)/[120/cancel169] × 25/144`
⇒ `119/120 × 25/144`
⇒ `595/3456`
APPEARS IN
संबंधित प्रश्न
If sin A = `3/4`, calculate cos A and tan A.
If cot θ =` 7/8` evaluate `((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ))`
In ΔABC, right angled at B. If tan A = `1/sqrt3` , find the value of
- sin A cos C + cos A sin C
- cos A cos C − sin A sin C
State whether the following are true or false. Justify your answer.
sec A = `12/5` for some value of angle A.
If 4 tan θ = 3, evaluate `((4sin theta - cos theta + 1)/(4sin theta + cos theta - 1))`
In the following, one of the six trigonometric ratios is given. Find the values of the other trigonometric ratios.
`tan theta = 8/15`
if `cot theta = 3/4` prove that `sqrt((sec theta - cosec theta)/(sec theta +cosec theta)) = 1/sqrt7`
Evaluate the following:
(cosec2 45° sec2 30°)(sin2 30° + 4 cot2 45° − sec2 60°)
Find the value of x in the following :
`sqrt3 sin x = cos x`
If `sqrt2 sin (60° – α) = 1` then α is ______.
3 sin² 20° – 2 tan² 45° + 3 sin² 70° is equal to ______.
If sin θ + sin² θ = 1, then cos² θ + cos4 θ = ______.
Given that sinα = `1/2` and cosβ = `1/2`, then the value of (α + β) is ______.
Prove that sec θ + tan θ = `cos θ/(1 - sin θ)`.
Proof: L.H.S. = sec θ + tan θ
= `1/square + square/square`
= `square/square` ......`(∵ sec θ = 1/square, tan θ = square/square)`
= `((1 + sin θ) square)/(cos θ square)` ......[Multiplying `square` with the numerator and denominator]
= `(1^2 - square)/(cos θ square)`
= `square/(cos θ square)`
= `cos θ/(1 - sin θ)` = R.H.S.
∴ L.H.S. = R.H.S.
∴ sec θ + tan θ = `cos θ/(1 - sin θ)`
Prove that: cot θ + tan θ = cosec θ·sec θ
Proof: L.H.S. = cot θ + tan θ
= `square/square + square/square` ......`[∵ cot θ = square/square, tan θ = square/square]`
= `(square + square)/(square xx square)` .....`[∵ square + square = 1]`
= `1/(square xx square)`
= `1/square xx 1/square`
= cosec θ·sec θ ......`[∵ "cosec" θ = 1/square, sec θ = 1/square]`
= R.H.S.
∴ L.H.S. = R.H.S.
∴ cot θ + tan θ = cosec·sec θ
If sin θ + cos θ = `sqrt(2)` then tan θ + cot θ = ______.
Let f(x) = sinx.cos3x and g(x) = cosx.sin3x, then the value of `7((f(π/7) + g(π/7))/(g((5π)/14) + f((5π)/14)))` is ______.
Let tan9° = `(1 - sqrt((sqrt(5)k)/m))k` where k = `sqrt(5) + 1` then m is equal to ______.
Evaluate: 5 cosec2 45° – 3 sin2 90° + 5 cos 0°.
