Advertisements
Advertisements
प्रश्न
If cot θ =` 7/8` evaluate `((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ))`
Advertisements
उत्तर १
Let us consider a right triangle ABC, right-angled at point B.
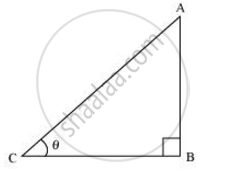
cot theta = `7/8`
If BC is 7k, then AB will be 8k, where k is a positive integer.
Applying Pythagoras theorem in ΔABC, we obtain
AC2 = AB2 + BC2
= (8k)2 + (7k)2
= 64k2 + 49k2
= 113k2
AC = `sqrt113k`
`sin theta = (8k)/sqrt(113k) = 8/sqrt(113)`
`cos theta = (7k)/sqrt(113k) = 7/sqrt113`
`((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ)) = (1-sin^2θ)/(1-cos^2θ)`
= `(1-(8/sqrt113)^2)/(1-(7/sqrt(113))^2)`
= `(1-64/113) /(1-49/113)`
= `(49/113)/(64/113)`
= `49/64`
उत्तर २
`cot theta = 7/8`
`((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ))`
= `(1 - sin^2 theta)/(1 - cos^2 theta)` ...[∵ (a + b) (a – b) = a2 − b2] a = 1, b = sin 𝜃
We know that sin 2𝜃 + cos2𝜃 = 1
1 − sin2𝜃 = cos2𝜃 = cos2𝜃
1 − cos2𝜃 = sin2 𝜃
= `(cos^2 theta)/(sin^2 theta)`
= `cot^2 theta`
= `(cot theta)^2`
= `[7/8]^2`
= `49/64`
संबंधित प्रश्न
Prove that `(sin "A" - 2sin^3 "A")/(2cos^3 "A" - cos "A") = tan "A"`
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sin A = 2/3`
In the following, one of the six trigonometric ratios is given. Find the values of the other trigonometric ratios.
tan θ = 11
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sin theta = 11/5`
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sec theta = 13/5`
If `tan theta = 1/sqrt7` `(cosec^2 theta - sec^2 theta)/(cosec^2 theta + sec^2 theta) = 3/4`
if `cos theta = 3/5`, find the value of `(sin theta - 1/(tan theta))/(2 tan theta)`
if `sin theta = 3/4` prove that `sqrt(cosec^2 theta - cot)/(sec^2 theta - 1) = sqrt7/3`
Evaluate the following
sin 45° sin 30° + cos 45° cos 30°
Evaluate the following
`2 sin^2 30^2 - 3 cos^2 45^2 + tan^2 60^@`
Evaluate the Following
cosec3 30° cos 60° tan3 45° sin2 90° sec2 45° cot 30°
If cosec θ - cot θ = `1/3`, the value of (cosec θ + cot θ) is ______.
3 sin² 20° – 2 tan² 45° + 3 sin² 70° is equal to ______.
If A and (2A – 45°) are acute angles such that sin A = cos (2A – 45°), then tan A is equal to ______.
A ladder rests against a vertical wall at an inclination α to the horizontal. Its foot is pulled away from the wall through a distance p so that its upper end slides a distance q down the wall and then the ladder makes an angle β to the horizontal. Show that `p/q = (cos β - cos α)/(sin α - sin β)`
In ΔABC, ∠ABC = 90° and ∠ACB = θ. Then write the ratios of sin θ and tan θ from the figure.
Prove that sec θ + tan θ = `cos θ/(1 - sin θ)`.
Proof: L.H.S. = sec θ + tan θ
= `1/square + square/square`
= `square/square` ......`(∵ sec θ = 1/square, tan θ = square/square)`
= `((1 + sin θ) square)/(cos θ square)` ......[Multiplying `square` with the numerator and denominator]
= `(1^2 - square)/(cos θ square)`
= `square/(cos θ square)`
= `cos θ/(1 - sin θ)` = R.H.S.
∴ L.H.S. = R.H.S.
∴ sec θ + tan θ = `cos θ/(1 - sin θ)`
Find the value of sin 45° + cos 45° + tan 45°.
In a right triangle PQR, right angled at Q. If tan P = `sqrt(3)`, then evaluate 2 sin P cos P.
