Advertisements
Advertisements
प्रश्न
If cot θ =` 7/8` evaluate `((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ))`
Advertisements
उत्तर १
Let us consider a right triangle ABC, right-angled at point B.
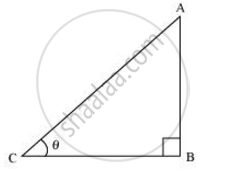
cot theta = `7/8`
If BC is 7k, then AB will be 8k, where k is a positive integer.
Applying Pythagoras theorem in ΔABC, we obtain
AC2 = AB2 + BC2
= (8k)2 + (7k)2
= 64k2 + 49k2
= 113k2
AC = `sqrt113k`
`sin theta = (8k)/sqrt(113k) = 8/sqrt(113)`
`cos theta = (7k)/sqrt(113k) = 7/sqrt113`
`((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ)) = (1-sin^2θ)/(1-cos^2θ)`
= `(1-(8/sqrt113)^2)/(1-(7/sqrt(113))^2)`
= `(1-64/113) /(1-49/113)`
= `(49/113)/(64/113)`
= `49/64`
उत्तर २
`cot theta = 7/8`
`((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ))`
= `(1 - sin^2 theta)/(1 - cos^2 theta)` ...[∵ (a + b) (a – b) = a2 − b2] a = 1, b = sin 𝜃
We know that sin 2𝜃 + cos2𝜃 = 1
1 − sin2𝜃 = cos2𝜃 = cos2𝜃
1 − cos2𝜃 = sin2 𝜃
= `(cos^2 theta)/(sin^2 theta)`
= `cot^2 theta`
= `(cot theta)^2`
= `[7/8]^2`
= `49/64`
संबंधित प्रश्न
In ΔABC right angled at B, AB = 24 cm, BC = 7 m. Determine:
sin A, cos A
If sin A = `3/4`, calculate cos A and tan A.
State whether the following are true or false. Justify your answer.
The value of tan A is always less than 1.
State whether the following are true or false. Justify your answer.
cot A is the product of cot and A.
State whether the following are true or false. Justify your answer.
sin θ = `4/3`, for some angle θ.
If `tan theta = a/b`, find the value of `(cos theta + sin theta)/(cos theta - sin theta)`
If `sin theta = a/b` find sec θ + tan θ in terms of a and b.
Evaluate the following
sin 45° sin 30° + cos 45° cos 30°
Evaluate the following
sin2 30° + sin2 45° + sin2 60° + sin2 90°
Find the value of x in the following :
`2 sin x/2 = 1`
Find the value of x in each of the following :
cos x = cos 60º cos 30º + sin 60º sin 30º
`(1 + tan^2 "A")/(1 + cot^2 "A")` is equal to ______.
If sin 2A = `1/2` tan² 45° where A is an acute angle, then the value of A is ______.
`(sin theta)/(1 + cos theta)` is ______.
If 4 tanθ = 3, then `((4 sintheta - costheta)/(4sintheta + costheta))` is equal to ______.
In ΔABC, ∠ABC = 90° and ∠ACB = θ. Then write the ratios of sin θ and tan θ from the figure.
Prove that sec θ + tan θ = `cos θ/(1 - sin θ)`.
Proof: L.H.S. = sec θ + tan θ
= `1/square + square/square`
= `square/square` ......`(∵ sec θ = 1/square, tan θ = square/square)`
= `((1 + sin θ) square)/(cos θ square)` ......[Multiplying `square` with the numerator and denominator]
= `(1^2 - square)/(cos θ square)`
= `square/(cos θ square)`
= `cos θ/(1 - sin θ)` = R.H.S.
∴ L.H.S. = R.H.S.
∴ sec θ + tan θ = `cos θ/(1 - sin θ)`
Find will be the value of cos 90° + sin 90°.
Let f(x) = sinx.cos3x and g(x) = cosx.sin3x, then the value of `7((f(π/7) + g(π/7))/(g((5π)/14) + f((5π)/14)))` is ______.
The maximum value of the expression 5cosα + 12sinα – 8 is equal to ______.
