Advertisements
Advertisements
प्रश्न
Given sec θ = `13/12`, calculate all other trigonometric ratios.
उत्तर
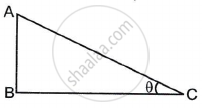
Let ΔABC be a right-angled triangle, right angled at point B.

It is given that:
sec θ = `"hypotenuse"/"side adjacent to ∠θ" = "AC"/"AB" = 13/12`
Let AC = 13k and AB = 12k, where k is a positive integer.
Applying pythagoras theorem in Δ ABC, we obtain:
AC2 = AB2 + BC2
BC2 = AC2 - AB2
BC2 = (13k)2 - (12k)2
BC2 = 169 k2 - 144 k2
BC2 = 25k2
BC = 5k
sin θ = `("side opposite to ∠θ")/("hypotenuse") = ("BC")/("AC") = 5/13`
cos θ = `("side adjacent to ∠θ")/("hypotenuse") = ("AB")/("AC") = 12/13`
tan θ = `("side opposite to ∠θ")/("side adjacent to ∠θ") = "(BC)"/"(AB)" = 5/12`
cot θ = `("side adjacent to ∠θ")/("side opposite to ∠θ") = ("AB")/("BC") = 12/5`
cosec θ = `("hypotenuse")/("side opposite to ∠θ") = ("AC")/("BC") = 13/5`
APPEARS IN
संबंधित प्रश्न
If cot θ =` 7/8` evaluate `((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ))`
If cot θ = `7/8`, evaluate cot2 θ.
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`cos A = 4/5`
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`cos theta = 7/25`
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sec theta = 13/5`
In the following, one of the six trigonometric ratios is given. Find the values of the other trigonometric ratios.
`cos theta = 12/2`
If 3 cot θ = 2, find the value of `(4sin theta - 3 cos theta)/(2 sin theta + 6cos theta)`.
If `tan theta = 1/sqrt7` `(cosec^2 theta - sec^2 theta)/(cosec^2 theta + sec^2 theta) = 3/4`
Evaluate the following:
(cosec2 45° sec2 30°)(sin2 30° + 4 cot2 45° − sec2 60°)
Evaluate the Following
cosec3 30° cos 60° tan3 45° sin2 90° sec2 45° cot 30°
Evaluate the Following
`(sin 30^@ - sin 90^2 + 2 cos 0^@)/(tan 30^@ tan 60^@)`
If sin (A − B) = sin A cos B − cos A sin B and cos (A − B) = cos A cos B + sin A sin B, find the values of sin 15° and cos 15°.
If cos A + cos² A = 1, then sin² A + sin4 A is equal to ______.
If cos (81 + θ)° = sin`("k"/3 - theta)^circ` where θ is an acute angle, then the value of k is ______.
In ΔABC, ∠ABC = 90° and ∠ACB = θ. Then write the ratios of sin θ and tan θ from the figure.
If sec θ = `1/2`, what will be the value of cos θ?
If cosec θ = `("p" + "q")/("p" - "q")` (p ≠ q ≠ 0), then `|cot(π/4 + θ/2)|` is equal to ______.
If θ is an acute angle and sin θ = cos θ, find the value of tan2 θ + cot2 θ – 2.
Evaluate 2 sec2 θ + 3 cosec2 θ – 2 sin θ cos θ if θ = 45°.
