Advertisements
Advertisements
प्रश्न
In a triangle ABC, D is the mid-point of side AC such that BD = `1/2` AC. Show that ∠ABC is a right angle.
Advertisements
उत्तर
Given: In ΔABC, D is the mid-point of AC i.e., AD = CD such that BD = `1/2` AC.
To show: ∠ABC = 90°
Proof: We have BD = `1/2` AC ...(i)
Since, D is the mid-point of AC.
∴ AD = CD = `1/2` AC ...(ii)
From equations (i) and (ii),
AD = CD = BD
In ΔDAB, AD = BD ...[Proved above]
∴ ∠ABD = ∠BAD ...(iii) [Angles opposite to equal sides are equal]
In ΔDBC, BD = CD ...[Proved above]
∴ ∠BCD = ∠CBD ...(iv) [Angles opposite to equal sides are equal]
In ΔABC, ∠ABC + ∠BAC + ∠ACB = 180° ...[By angle sum property of a triangle]
⇒ ∠ABC + ∠BAD + ∠DCB = 180°
⇒ ∠ABC + ∠ABD + ∠CBD = 180° ...[From equations (iii) and (iv)]
⇒ ∠ABC + ∠ABC = 180°
⇒ 2∠ABC = 180°
⇒ ∠ABC = 90°
APPEARS IN
संबंधित प्रश्न
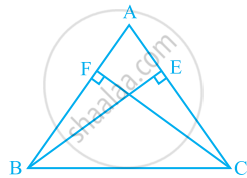
ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively (see the given figure). Show that these altitudes are equal.
Find the measure of each exterior angle of an equilateral triangle.
In Fig. 10.23, PQRS is a square and SRT is an equilateral triangle. Prove that
(i) PT = QT (ii) ∠TQR = 15°
Find the measure of each exterior angle of an equilateral triangle.
BD and CE are bisectors of ∠B and ∠C of an isosceles ΔABC with AB = AC. Prove that BD = CE.
If the bisector of the exterior vertical angle of a triangle be parallel to the base. Show that the triangle is isosceles.
ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
Which of the following statements are true (T) and which are false (F):
Angles opposite to equal sides of a triangle are equal
Which of the following statements are true (T) and which are false (F):
The two altitudes corresponding to two equal sides of a triangle need not be equal.
In ΔABC, if ∠A = 40° and ∠B = 60°. Determine the longest and shortest sides of the triangle.
Which of the following statements are true (T) and which are false (F)?
If two angles of a triangle are unequal, then the greater angle has the larger side opposite to it.
Fill in the blank to make the following statement true.
If two sides of a triangle are unequal, then the larger side has .... angle opposite to it.
Write the sum of the angles of an obtuse triangle.
Line segments AB and CD intersect at O such that AC || DB. If ∠CAB = 45° and ∠CDB = 55°, then ∠BOD =
In the given figure, if EC || AB, ∠ECD = 70° and ∠BDO = 20°, then ∠OBD is
In the given figure, x + y =
The side BC of ΔABC is produced to a point D. The bisector of ∠A meets side BC in L. If ∠ABC = 30° and ∠ACD = 115°, then ∠ALC = ______.
Which of the following correctly describes the given triangle?
In ∆PQR, ∠P = 70° and ∠R = 30°. Which side of this triangle is the longest? Give reason for your answer.
