Advertisements
Advertisements
प्रश्न
In Fig. 8.78, there are two concentric circles with centre O. PRT and PQS are tangents to the inner circle from a point P lying on the outer circle. If PR = 5 cm, find the length of PS.

उत्तर
Given that PR = 5 cm.
PR and PQ are the tangents to the inner circle so,
PR = PQ = 5 cm (Tangents drawn from an external point to the circle are equal)
Now draw a perpendicular from the centre O to the tangent PS.
PS is the chord of the inner circle. we know that the perpendicular drawn
from the centre of the circle to the chord bisects the chord. So, PQ = QS = 5 cm
PS = PQ + QS = 5 cm + 5 cm = 10 cm
APPEARS IN
संबंधित प्रश्न
Fill in the blank:
A point whose distance from the centre of a circle is greater than its radius lies in ..................... of the circle.
If the area of a circle is equal to sum of the areas of two circles of diameters 10 cm and 24 cm, then the diameter of the larger circle (in cm) is:
A chord of length 14 cm is at a distance of 6 cm from the centre of a circle. The length of another chord at a distance of 2 cm from the centre of the circle is
In the given figure, OQ : PQ = 3.4 and perimeter of Δ POQ = 60 cm. Determine PQ, QR and OP.

In the given figure, PA and PB are tangents to the circle from an external point P. CD is another tangent touching the circle at Q. If PA = 12 cm, QC = QD = 3 cm, then find PC + PD.
State, if the following statement is true or false:
If the end points A and B of the line segment lie on the circumference of a circle, AB is a diameter.
A point A is 26 cm away from the centre of a circle and the length of the tangent drawn from A to the circle is 24 cm. Find the radius of the circle.

In the given figure, O is the centre of the circle. Name all chords of the circle.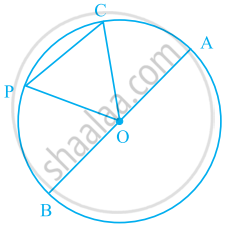
From the figure, identify two points in the interior.

In the given figure, O is the centre of the circle. If ∠ AOB = 145°, then find the value of x.

