Advertisements
Advertisements
प्रश्न
In figure , ABC is an isosceles triangle inscribed in a circle with centre O such that AB = AC = 13 cm and BC = 10 cm .Find the radius of the circle.

उत्तर

Since ABC is an isosceles triangle, AOO is the perpendicular bisector of BC.
In triangle ADC, by Pythagoras theorem we have
AD2 = AC2 - DC2 = 132 - 52 = 169 - 25 = 144
⇒ AD = 12 cm ⇒ AO + OD = 12 ⇒ AO = 12 - x ...(Assuming OD = x cm)
Again in triangle OBD,
BO2 = BD2 + OD2 = 25 + x2 ..(As BD = 5 cm)
⇒ (12 - x)2 = 25 + x2 ..(As AO = BO = radius)
⇒ 144 + x2 - 24 x = 25 + x2
⇒ -24 x = 25 - 144 = - 119
⇒ x = 4.96 cm
⇒ AO = 12 - 4. 96 = 7 .04 cm
APPEARS IN
संबंधित प्रश्न
If the sides of a quadrilateral ABCD touch a circle, prove that : AB + CD = BC + AD.

In quadrilateral ABCD; angles D = 90°, BC = 38 cm and DC = 25 cm. A circle is inscribed in this quadrilateral which touches AB at point Q such that QB = 27 cm, Find the radius of the circle.
In the given figure, PT touches the circle with centre O at point R. Diameter SQ is produced to meet the tangent TR at P. Given ∠SPR = x° and ∠QRP = y°;
Prove that:
- ∠ORS = y°
- write an expression connecting x and y.
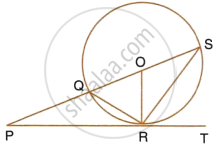
PT is a tangent to the circle at T. If ∠ABC = 70° and ∠ACB = 50°; calculate:
- ∠CBT
- ∠BAT
- ∠APT

If PQ is a tangent to the circle at R; calculate:
- ∠PRS,
- ∠ROT.
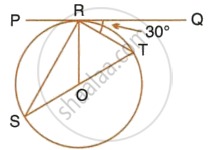
Given O is the centre of the circle and angle TRQ = 30°.
AB is the diameter and AC is a chord of a circle with centre O such that angle BAC = 30°. The tangent to the circle at C intersects AB produced in D. show that BC = BD.
ABC is a right triangle with angle B = 90°, A circle with BC as diameter meets hypotenuse AC at point D. Prove that: AC × AD = AB2
In the figure, PM is a tangent to the circle and PA = AM. Prove that:
(i) Δ PMB is isosceles
(ii) PA x PB = MB2

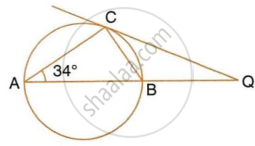
In the given figure, AB is the diameter. The tangent at C meets AB produced at Q. If ∠CAB = 34°, find:
- ∠CBA
- ∠CQB
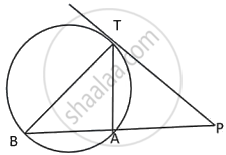
In the given figure PT is a tangent to the circle. Chord BA produced meets the tangent PT at P.
Given PT = 20 cm and PA = 16 cm.
- Prove ΔPTB ~ ΔPAT
- Find the length of AB.