Advertisements
Advertisements
प्रश्न
In figure , ABC is an isosceles triangle inscribed in a circle with centre O such that AB = AC = 13 cm and BC = 10 cm .Find the radius of the circle.

उत्तर

Since ABC is an isosceles triangle, AOO is the perpendicular bisector of BC.
In triangle ADC, by Pythagoras theorem we have
AD2 = AC2 - DC2 = 132 - 52 = 169 - 25 = 144
⇒ AD = 12 cm ⇒ AO + OD = 12 ⇒ AO = 12 - x ...(Assuming OD = x cm)
Again in triangle OBD,
BO2 = BD2 + OD2 = 25 + x2 ..(As BD = 5 cm)
⇒ (12 - x)2 = 25 + x2 ..(As AO = BO = radius)
⇒ 144 + x2 - 24 x = 25 + x2
⇒ -24 x = 25 - 144 = - 119
⇒ x = 4.96 cm
⇒ AO = 12 - 4. 96 = 7 .04 cm
APPEARS IN
संबंधित प्रश्न
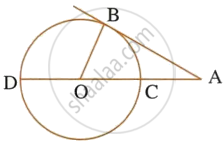
In the given figure, O is the center of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm, calculate the radius of the circle.
From a point P outside a circle, with centre O, tangents PA and PB are drawn. Prove that:
∠AOP = ∠BOP
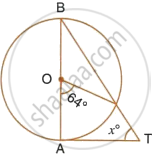
In the given figure, AB is the diameter of the circle, with centre O, and AT is the tangent. Calculate the numerical value of x.
In the given figure, O is the centre of the circumcircle ABC. Tangents at A and C intersect at P. Given angle AOB = 140° and angle APC = 80°; find the angle BAC.

AB is the diameter and AC is a chord of a circle with centre O such that angle BAC = 30°. The tangent to the circle at C intersects AB produced in D. show that BC = BD.
ABC is a right triangle with angle B = 90°, A circle with BC as diameter meets hypotenuse AC at point D. Prove that: AC × AD = AB2
In the following figure, PQ and PR are tangents to the circle, with centre O. If ∠ QPR = 60° , calculate:
∠ OQR
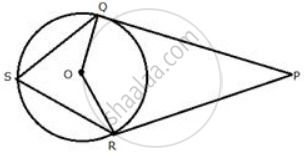
In the following figure, PQ and PR are tangents to the circle, with centre O. If ∠ QPR = 60° , calculate:
∠ QSR
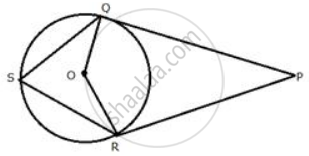
In the given figure, PT touches a circle with centre O at R. Diameter SQ when produced to meet the tangent PT at P. If ∠SPR = x° and ∠QRP = y°; Show that x° + 2y° = 90°

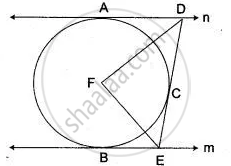
In Fig. l and m are two parallel tangents at A and B. The tangent at C makes an intercept DE between n and m. Prove that ∠ DFE = 90°