Question

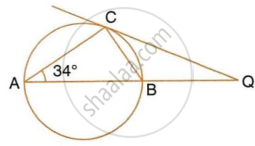
In the given figure, AB is the diameter. The tangent at C meets AB produced at Q. If ∠CAB = 34°, find:

1. ∠CBA
2. ∠CQB

Answer 1

i. AB is a diameter.

∴ ∠ACB = 90°

The angle in a semicircle is the right angle.

∴ In ΔACB,

∠A + ∠C + ∠B = 180°

34° + 90° + ∠B = 180°

∠B = 180° – (34° + 90°)

∠B = 180° – 124°

∠B = 56°

ii. Now,

CQ is tangent.

∴ ∠QCB = ∠CAB  ...(Alternate segment angle)

∴ ∠QCB = 34°

And ∠CBQ = 180° – ∠CBA

∠CBQ = 180° – 56° = 124°

∴ ∠CQA = 180° – (∠QCB + ∠CBQ)

∠CQA = 180° – (34° + 124°)

∠CQA = 180° – 158° = 22°.