Advertisements
Advertisements
प्रश्न
In figure, two line segments AC and BD intersect each other at the point P such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30°. Then, ∠PBA is equal to ______.
विकल्प
50°
30°
60°
100°
उत्तर
In figure, two line segments AC and BD intersect each other at the point P such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30°. Then, ∠PBA is equal to 100°.
Explanation:

From ∆APB and ∆CPD,
∠APB = ∠CPD = 50° ...(Since they are vertically opposite angles)
`("AP")/("PD") = 6/5` ...(i)
Also, `("BP")/("CP") = 3/2.5`
Or `("BP")/("CP") = 6/5` ...(ii)
From equations (i) and (ii),
We get,
`("AP")/("PD") = ("BP")/("CP")`
So, ∆APB ∼ ∆DPC ...[Using SAS similarity criterion]
∴ ∠A = ∠D = 30° ...[Since, corresponding angles of similar triangles]
Since, Sum of angles of a triangle = 180°,
In ∆APB,
∠A + ∠B + ∠APB = 180°
So, 30° + ∠B + 50° = 180°
Then, ∠B = 180° – (50° + 30°)
∠B = 180° – 80° = 100°
Therefore, ∠PBA = 100°
APPEARS IN
संबंधित प्रश्न
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:

In the following figure, altitudes AD and CE of ΔABC intersect each other at the point P. Show that:

ΔPDC ∼ ΔBEC
CD and GH are, respectively, the bisectors of ∠ACB and ∠EGF such that D and H lie on sides AB and FE of ΔABC and ΔEFG, respectively. If ΔABC ~ ΔFEG, Show that
- `("CD")/("GH") = ("AC")/("FG")`
- ΔDCB ~ ΔHGE
- ΔDCA ~ ΔHGF
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR, prove that `("AB")/("PQ") = ("AD")/("PM")`.
In the given figure, two chords AB and CD intersect each other at the point P. prove that:
(i) ΔAPC ∼ ΔDPB
(ii) AP.BP = CP.DP
In a right angled triangle with sides a and b and hypotenuse c, the altitude drawn on the hypotenuse is x. Prove that ab = cx.
In the given figure, seg AC and seg BD intersect each other in point P and `"AP"/"CP" = "BP"/"DP"`. Prove that, ∆ABP ~ ∆CDP.

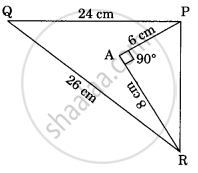
In the given figure, PQ = 24 cm, QR = 26 cm ∠PAR = 90°, PA = 6 cm, and AR = 8 cm, the degree measure of ∠QPR is ______.
Areas of two similar triangles are 36 cm2 and 100 cm2. If the length of a side of the larger triangle is 20 cm, find the length of the corresponding side of the smaller triangle.
Which of the following is NOT a similarity criterion of traingles?
