Advertisements
Advertisements
рдкреНрд░рд╢реНрди
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`cosec theta = sqrt10`
рдЙрддреНрддрд░
consider a right-angled Δle ABC, we get
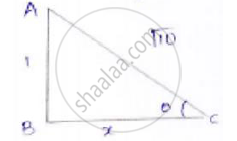
Let x be the adjacent side.
By applying Pythagoras theorem
ЁЭР┤ЁЭР╢2 = ЁЭР┤ЁЭР╡2 + ЁЭР╡ЁЭР╢2
`(sqrt10)^2 = 1^2 + x^2`
x2 = 10 − 1 = 9
x = 3
`sin theta = 1/cosec theta = 1/sqrt10`
`cos theta = "adjacent"/"hypotenuse" = 3/sqrt10`
`tan theta = "opposite sides"/"adjacebt side" = 1/3`
`sec theta = 1/cos theta = sqrt10/3`
`cot theta = 1/tan theta = (1/1)/3 = 3`
APPEARS IN
рд╕рдВрдмрдВрдзрд┐рдд рдкреНрд░рд╢реНрди
State whether the following are true or false. Justify your answer.
sec A = `12/5` for some value of angle A.
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sin theta = 11/5`
Find the value of x in the following :
`2sin 3x = sqrt3`
Find the value of x in the following :
`sqrt3 tan 2x = cos 60^@ + sin45^@ cos 45^@`
sin (45° + θ) – cos (45° – θ) is equal to ______.
Prove the following:
If tan A = `3/4`, then sinA cosA = `12/25`
Find the value of sin 0° + cos 0° + tan 0° + sec 0°.
Find will be the value of cos 90° + sin 90°.
`sqrt(3)` cos2A + `sqrt(3)` sin2A is equal to ______.
If sin θ + cos θ = `sqrt(2)` then tan θ + cot θ = ______.
